a tuning study
© 1998 by Joe Monzo
written 1998-4-9, revised 1998-10-29
The "Jimi Hendrix Chord" (Dominant 7th, sharp 9th)
(you're listening to one excerpt of it)
This piece is a virtuoso étude for the regular 12-EQ Guitar. The major
challenge is the finger independence needed to fret a chord and bend each
of the four fingered strings separately by the proper amount to acheive
a particular just-intonation chord.
It was inspired by a
debate I had with Paul Erlich
(via the Tuning Forum)
over which ratios would most likely be implied
by the "sharp 9th" [2(3/12) = 3.00 semitones] of the 12-EQ chord Jimi
played, and with which each phrase
of my piece begins.
I had originally made a statement that
Jimi Hendrix deserves the credit for bringing 19/16 [191 = 2.98 semitones] decisively into
the harmonic language of rock. Erlich pointed out that, because Jimi played the
chord on guitar with the regular 12-EQ frets, I
had neglected to take into account the fact that the "7th" of
the 12-EQ chord [2(10/12) = 10.00 semitones] was 31 cents too sharp to
represent 7/4 [71 = 9.69 semitones]. He
suggested that if Jimi bent the
two lowest notes (played with the stronger fingers) upward, the best rational
interpretation that could be given to the top two 12-EQ notes (played by
the weaker fingers, and with no bending) would
be to call them a 7/4 and a 7/6 [3-1 71 = 2.67 semitones],
his main point being that the interval between the top
two notes in 12-EQ, a "perfect 4th" [2(5/12) = 5.00 semitones], was only 2 cents
larger than the interval between 7/4 and 7/6: a 4/3 [= 4.98 semitones].
Interestingly, though, 19/16 [= 2.98 semitones] is only 2 cents
smaller than the interval between the original bass note (before
bending) and the 12-EQ "sharp 9th" [2(3/12) = 3.00 semitones]. Thus,
while I basically came
to agree with Erlich's suggestion, it seemed possible to
me that a lingering memory of the original bass note may
partially force one to hear the "sharp 9th" as being able to represent 19/16.
Also, I feel that the 19/16 has a funkier, bluesier sound
than 7/6, and I like it. Thus,
the idea for this piece.
(For those interested in reading the entire discussion, it
can be found
here
on Drew Skyfyre's site. It's quite long.)
Here's the excerpt the page opened with.
This is a graph of the pitches used in the chord progression
which cycles around each time:
in 19-Limit just-intonation.
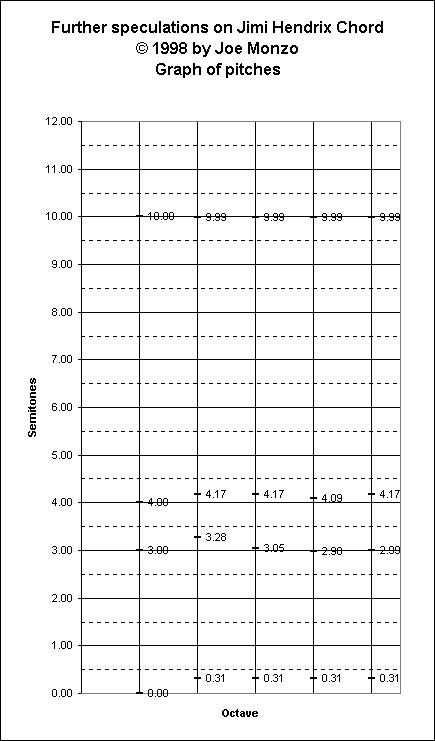
The excerpt in the audio example analyzed below uses only phrases 17 to 26 of the first section of the piece.
All of them start as 12-EQ: 20 : 2(4/12) : 2(10/12) : 2(3/12) then bend upwards into a JI chord with the proportions 4:10:14:19, on the following "roots" which get successively higher (the ones ending with ++ are particularly smooth to my ears):
| phrase # | chord root | ~cents | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2(10/12) 7-1 | [i.e.,
in this just chord, the 7-identity
is the un-bent 12-EQ "7th", 2(10/12)] 2 | 5-1 71 11-1 | ++ | 3 | 3-1 7-1 13-1 1391 | 4 | 7-1 131 171 31-1 | 5 | 7-1 23-1 411 | 6 | 7-1 19-1 2711 | 7 | 33 53-1 | 8 | 3-1 5-1 7-1 1071 | 9 | 7-1 67-1 2391 | 10 | 31 7-1 111 37-1 | 11 | 7-1 11-1 1571 | 12 | 3-1 131 17-1 | ++ | 13 | 32 7-1 231 29-1 | 14 | 5-1 7-1 13-1 291 | 15 | 3-2 7-1 2571 | 16 | 31 7-1 471 79-1 | 17 | 52 7-2 | 35.0 | ++ | (audio example starts here) | 18 | 5-1 7-1 111 131 | 36.7 | ++ | 19 | 3-1 7-1 11-1 591 | 37.1 | 20 | 31 7-1 13-1 311 | 37.6 | 21 | 7-1 171 19-1 | 38.6 | ++ | 22 | 3-1 7-1 431 | 40.7 | 23 | 7-1 17-1 611 | 43.1 | 24 | 32 5-1 7-1 | 48.8 | ++ | 25 | 7-1 291 | 60.8 | 26 | 3-1 7-1 111 | 80.5 | |
Updated: 2002.2.11. 2000.8.23, 1999.4.14
|
or try some definitions. |
I welcome
feedback about this webpage:
|