An equal temperament with an integer number of notes per octave is consistent with JI through some odd limit if a complete chord of that limit is constructed in that equal temperament in the same way no matter which intervals are approximated.
If for all odd integers a, b, c such that 1 <= a < b < c <= n, the ET's best approximation of b /a plus the ET's best approximation of c /b equals the ET's best approximation of c /a, then the ET is consistent in the n-limit.
For example, the smallest ETs consistent in the 11, 13, 15, and 17-limits are 22, 26, 29, and 58-tET, respectively.
Consistency may be defined for non-octave equal temperaments but then even as well as odd numbers must be considered, and the consistency will be through an "integer limit" rather than an odd limit.
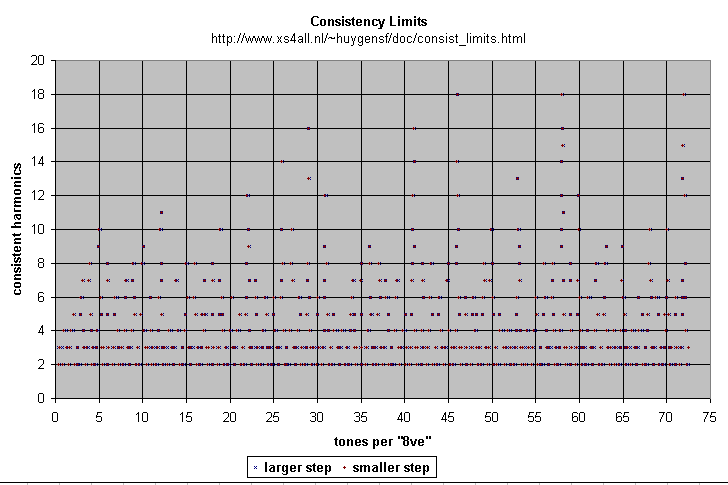
The data for case are tabulated at http://www.xs4all.nl/~huygensf/doc/consist_limits.html and http://www.xs4all.nl/~huygensf/doc/cons_limit_bounds.html. The ordinary, odd-limit consistency of an integer ET can be read from this table as well: it is the largest odd number not exceeding the integer limit.
[from Paul Erlich, "Tuning, Tonality, and Twenty-Two Tone Temperament", Xenharmonikon 17, footnote 8]
The data for ETs thru 73 are plotted on the following chart:
see Patrick Ozzard-Low's 21st Century Orchestral Instruments for a more in-depth exploration of consistency.
see also unique
Updated: 2002.1.12