An interval of measurement with a size of approximately 116.7 cents.
The term honors the name of George Secor, original discoverer of the MIRACLE tunings. This interval (and other close approximations to it) is particularly important as the generator of the MIRACLE tunings.
The minimax optimum value for the secor, based on its ability to generate temperaments which contain many close approximations of JI ratios, is (18/5)^(1/19) = ~116.7155940982074 cents. This satisfies the property that Secor mentions in his Xenharmonik˘n article, that all the primary 11-limit ratios (aka 11-limit consonances) will be approximated with an error not greater than 3.32 cents.
The commonly-used
EDOs
which most closely approximate secor-generated tunings
are 31-, 41-, and 72-EDO. Their secors are:
EDO ratio ~cents
31 2(3/31) 116.13 cents
41 2(4/41) 117.07 cents
72 2(7/72) 116.67 cents
Below is a graph showing the closest EDO approximations, those closest to "0" giving the best approximation. Above 72, the best approximations are given by 185-, 257-, and 329-EDO.
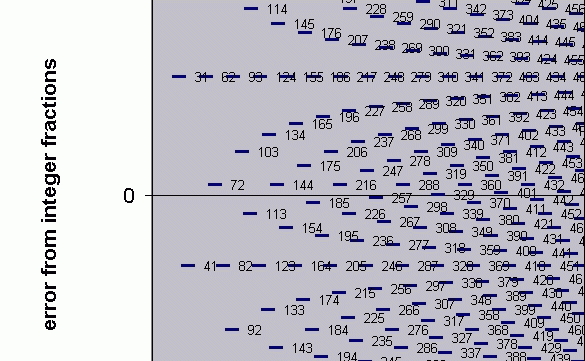
The closest low-integer rational approximation
of the secor is 46:43 (= ~116.7566416 cents),
which is nearly identical to 2(18/185).
REFERENCE
Secor, George.
A
New Look at the Partch Monophonic Fabric, originally
published in Xenharmonik˘n 3.
A footnote by Paul Erlich on minimax:
Minimax simply means "minimizing the maximum error". The lowest value of largest error is acheived there. For example, 1/4-comma meantone is the "minimax" meantone with respect to the 5-limit, as all 5-limit intervals are approximated with a maximum error of 1/4-comma, and no other meantone can improve on that (see the "historical meantones" chart at the bottom of Joe's meantone definition page to see why).
[from Joe Monzo, JustMusic: A New Harmony, with helpful additions from Paul Erlich]
Updated: 2001.11.16