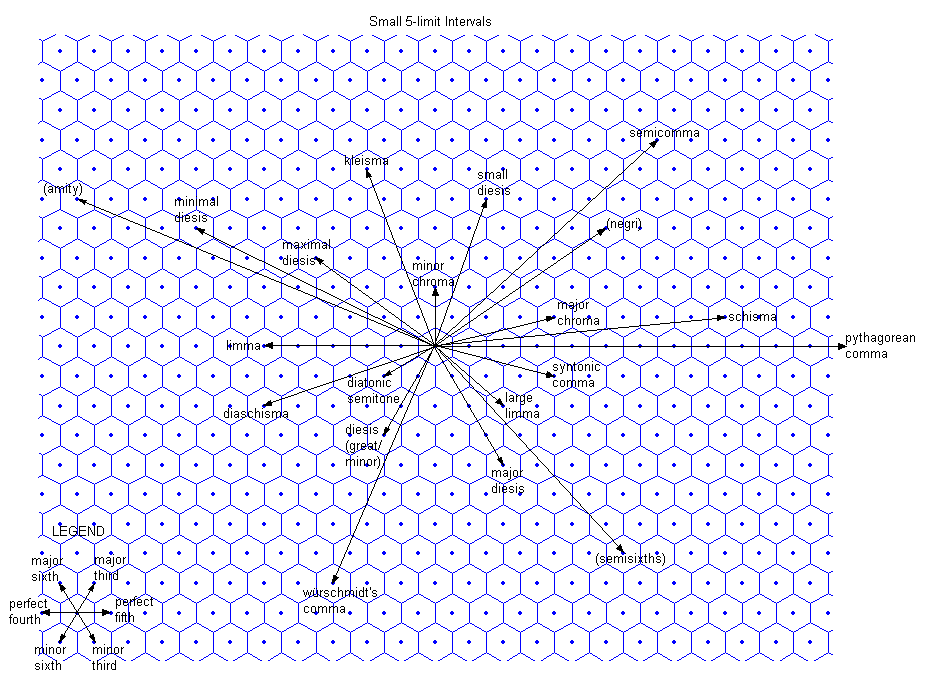
- the syntonic comma, designated in [3 5] prime-factor vector-notation by the exponents [4 -1], (making 12edo function as a meantone, specifically 1/11-comma meantone),
- the diaschisma [-4 -2],
- the diesis [0 -3], and also
- the skhisma [8 1],
- the Pythagorean comma [12 0],
A type of lattice diagram used to represent equal-temperaments, in which the basic prime-axis interval in each dimension of the lattice (i.e., 1 "step"of that dimension) is rounded to its nearest EDO-degree representation, then those basic values are added to and subtracted from each other, to fill out all the other EDO-degree numbers on the lattice.
This process results in a periodic tiling of periodicity-blocks across the lattice, each block containing all or some of the degrees of the EDO (depending on how big the EDO is and whether or not it is a multiple of a lower-cardinality EDO), in a pattern which thus is not random, but which at first glance appears to be random, and which in the case of a 2-dimensional lattice (like the 5-limit ones shown on this page) resembles the cards used to play the popular gambling game of bingo. As far as i know, the application of the name "bingo-card" was the idea of Paul Erlich, and the earliest author i know to have used them prominently was James McCartney.
Most often, the resulting degree-numbers in a bingo-card lattice are slightly different from those obtained by merely finding the closest EDO-degree which can approximately represent the JI ratios. The amount of error between these two methods depends on the cardinality of the EDO under consideration, and also on the size and shape of the lattice, as different EDOs approximate JI intervals in different ways. The amount of error can be seen occurring on the bingo-card lattice in patterns, and these patterns echo, more broadly, the patterns in my lattices of the nearest EDO approximation to JI. (For an example of an EDO without appreciable error, see 53edo below.)
The utility of the bingo-card lattices lies in their ability to show the "vanishing commas", i.e., the small intervals which are tempered out in a particular EDO. Below is a lattice showing many of these intervals, with most of the more important ones labeled with the names in common use among tuning theorists c. 2000.
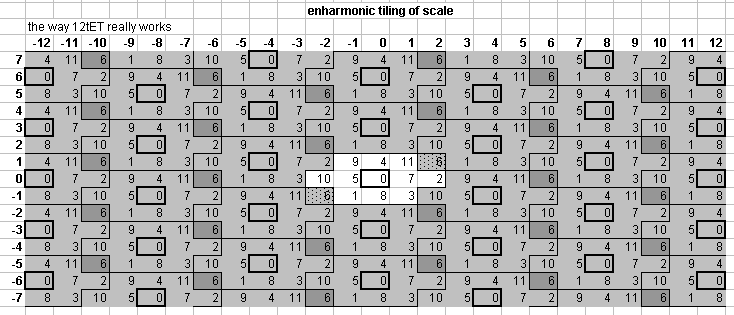
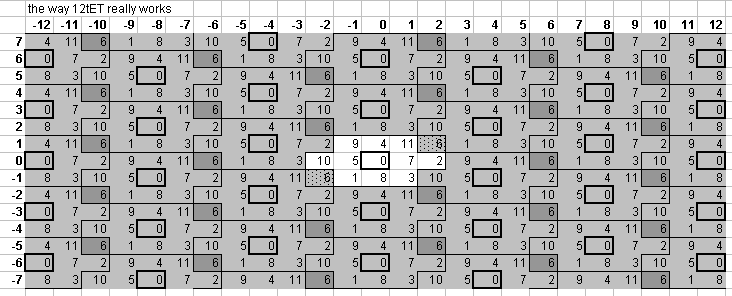
The bingo-card lattice for 12edo will be explained in detail as an example; the technique used in creating that lattice applies for all the others.
12edo
These lattices all represent equal-tempered approximations to an arbitrarily large subset of the theoretically infinite 5-limit just-intonation tuning. They assume "8ve"-equivalence, and thus do not have any representation of prime-factor 2, and thus are 2- rather than 3-dimensional. The whole system is an Euler-genus which can be described compactly as 3(-12...+12)*5(-7...+7) The row of numbers across the top of each lattice designates the exponents of prime-factor 3, and the column of numbers along the left edge designate the exponents of prime-factor 5.
The 5-limit "vanishing commas" (within the arbitrary limits described above) in 12edo can be seen on this "bingo-card" lattice:

Looking near the center of the lattice, it can be seen that the 3/2 ratio, designating the musical interval of the "perfect 5th", written in [3 5]-prime-factor vector notation as [1 0], is represented by 7 degrees of 12edo; the 5/4 ratio, designating the interval of the "major 3rd", in vector format [0 1], is represented by 4 degrees of 12edo. All other degrees are obtained by adding or subtracting 7 mod 12 along the horizontal (i.e., 3) axis and by adding or subtracting 4 mod 12 along the vertical (i.e., 5) axis.
For example, starting at "7" in the [1 0] cell,
let's find the "major-2nd":
prime-factor vectors
2 3 5 ratios
[ 7/12] ≡ [1 0] 3/2 "perfect 5th"
+ [ 7/12] ≡ + [1 0] * 3/2 "perfect 5th"
--------- -------- -----
[14/12] ≡ [2 0] 9/4 "major 9th"
and since the lattice is considered to be 8ve-invariant, and so a "major 9th" is equivalent to a "major 2nd", then
just as 9/4 ≡ 9/8,
so 2( (14 mod 12) / 12) = 2(2/12),
and so 2(14/12) ≡ 2(2/12).
Thus, the 12edo degree "2", designating the interval 2(2/12), is placed in the cell representing 9/8 = [2 0].
For another, slightly more complicated example, let's fill
in the cell a "minor-3rd"
above the reference 1/1 = [0 0]. The "minor 3rd" is ratio 6/5,
which is 31 * 5-1, or a "perfect 5th" minus
a "major 3rd":
prime-factor vectors
2 3 5 ratios
[ 7/12] ≡ [1 0] 3/2 "perfect 5th"
- [ 4/12] ≡ - [0 1] ÷ 5/4 "major 3rd"
--------- -------- -----
[ 3/12] ≡ [1 -1] 6/5 "minor 3rd"
Thus, the 12edo degree "3" is placed in the cell representing 6/5 = [1 -1]. And so on.
Eventually (because of the incommensurability of prime-multiples), for every EDO, a point will be found where the just ratio is better represented by another EDO-degree different from the one found by this process of vector-addition. As an example, let's examine the 12edo representation of successive "major 3rds", which are the degree-numbers above the bold-outlined zero in the central column:
In the yellow cell representing [0 4], which designates 54,
the bingo-card lattice above has "4", meaning 4 degrees of
12edo (mathematically 2(4/12)),
derived from successive addition:
12edo cents interval ratio cents of ratio error of 12edo
0 0 prime (1me) 1/1 0 0
+ 4 + 400 major-3rd * 5/4 + 386.3137139
--- ------ -------- ---------------
4 400 major-3rd 5/4 386.3137139 + 13.68628614
+ 4 + 400 major-3rd * 5/4 + 386.3137139
--- ------ -------- ---------------
8 800 augmented-5th 25/16 772.6274277 + 27.37257227
+ 4 + 400 major-3rd * 5/4 + 386.3137139
--- ------ -------- ---------------
12 1200 augmented-7th 125/64 1158.941142
mod 12 - 1200 "8ve"-reduce * 1/2 - 1200
------ ------ -------- ---------------
0 0 125/128 -41.05885841 + 41.05885841
+ 4 + 400 major-3rd * 5/4 + 386.3137139
--- ------ -------- ---------------
4 400 doubly-augmented-2nd 625/512 345.2548555 + 54.74514454
compare with:
3 300 doubly-augmented-2nd 625/512 345.2548555 - 45.25485546
2(4/12) represents 54 with an error of +~54 3/4 cents. But the table shows that 3 degrees (= 2(3/12)) provides a closer approximation, with an error of -~45 1/4 cents. To find the fractional value that shows the nearest 12edo approximation, one may simply divide the cents-value by 100, to obtain ~3.452548555 Semitones, which does indeed show that 3 degrees is a closer approximation than 4.
Where a 12edo degree on the bingo-card lattice is different from the nearest 12edo approximation of the corresponding ratio in the just lattice, those lattice-cells are colored: yellow where the 12edo note is 1 degree too high, and blue where it is 1 degree too low. Thus, the cell just examined is yellow because its 12edo approximation to the "doubly-augmented-2nd" is 4 degrees of 12edo instead of the closer approximation of 3 degrees. The legend is as follows:

The cells outlined in black are all the instances of 2(0/12), which shows immediately which of the commas vanish (as per the graph given above), and thus the periodicity.
This lattice shows that 12edo tempers out a large number of small 5-limit intervals, including:
and others which can be found by adding together various combinations of the syntonic comma, diaschisma, and diesis.
12edo does not temper out the kleisma, porcupine comma, magic comma, semicomma, or ampersand's comma.
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 12edo:
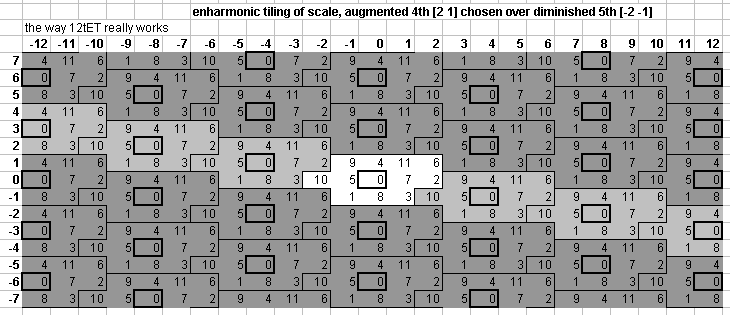
In this and all of the "tiling" lattices below, the objective was to outline the periodicity-block which forms the source-cell at the center of the lattice -- i.e., all ratios as close as possible, by the taxicab metric, to the 1/1 at the origin point.
in many cases one or more EDO representations of ratios may be the same distance from 1/1, and so they must belong to multiple tiles simultaneously.
... anyway, this is trivial, because the unison-vectors bounding the periodicity-block may be moved incrementally along either the 3- or 5-axis or both, so that the source periodicity-block encloses entirely and uniquely only the exact number specified by the cardinality of the EDO, without changing the essential nature of that particular periodicity-block other than to eliminate duplicate pitches at the boundaries.
Thus, in 12edo, we would obtain either a periodicity-block which includes the "augmented 4th" ("tritone") but not the "diminished 5th":
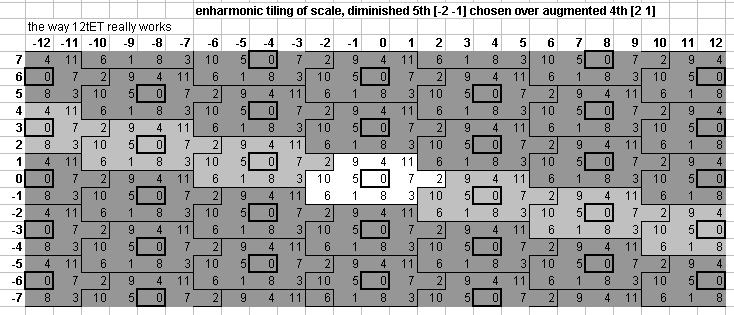
... or alternatively, one which includes the "diminished 5th" but not the "augmented 4th":
12edo is very often used as a pseudo-Pythagorean aristoxenean temperament, in a cycle with letter-names from Eb to G#. Below is a lattice showing this mapping, but this time substituting letter-names for degree-numbers for all those which would be found in the standard repertoire (degree-numbers for the rest), and showing enharmonic equivalents as darker shades of grey:

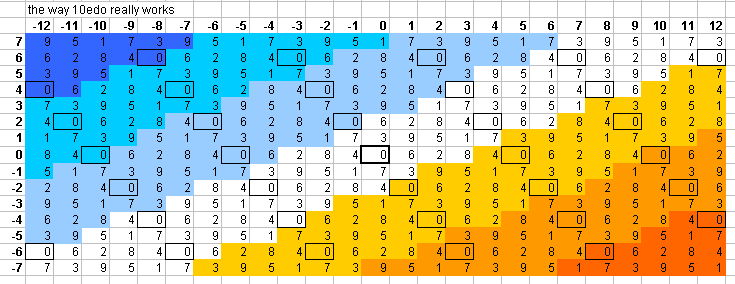
10edo
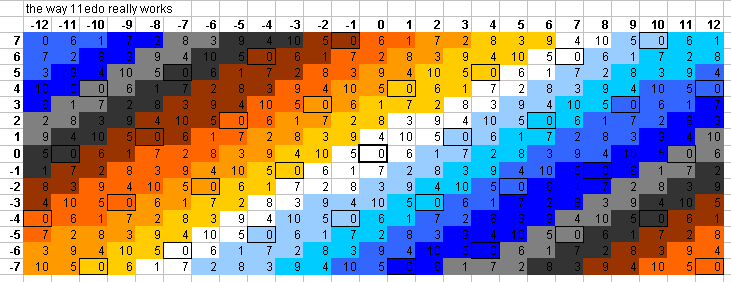
11edo
12edo
(see above)
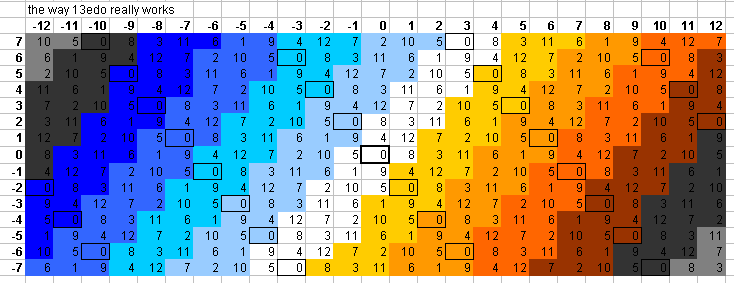
13edo
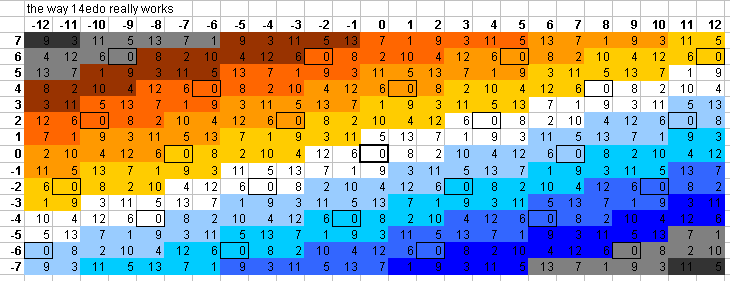
14edo
15edo
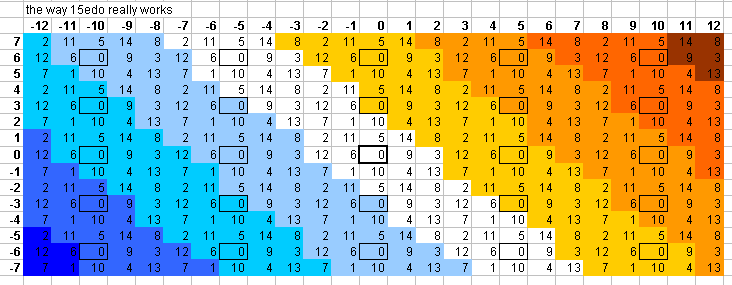
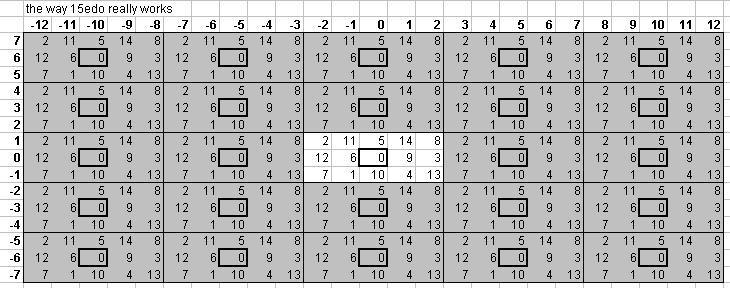
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 15edo:
16edo
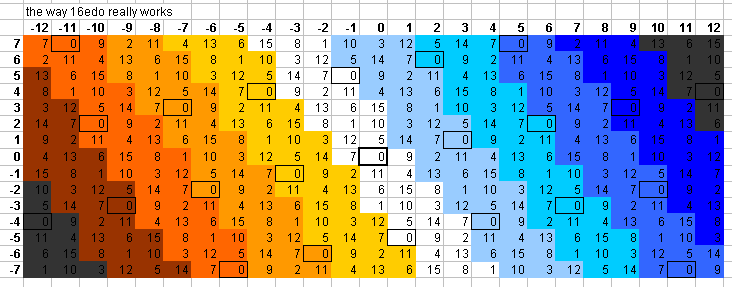
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 16edo:
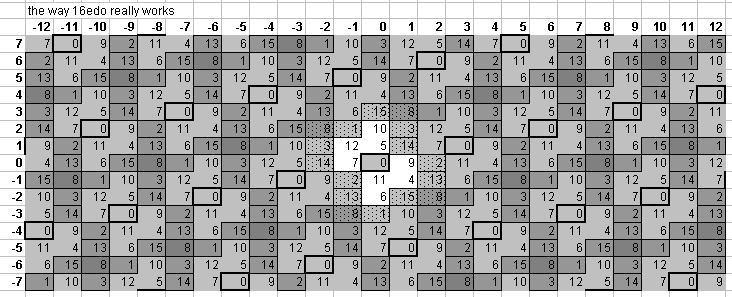
16edo tempers out the magic comma [-1 5] and also the rather large ratio 128/135 [3 1] (= ~92 cents) called "larger limma" by Ellis and "mean semitone" by Rameau, and their derivatives.
16edo does not temper out the syntonic comma, kleisma, skhisma, diaschisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, semicomma, or ampersand's comma.
17edo
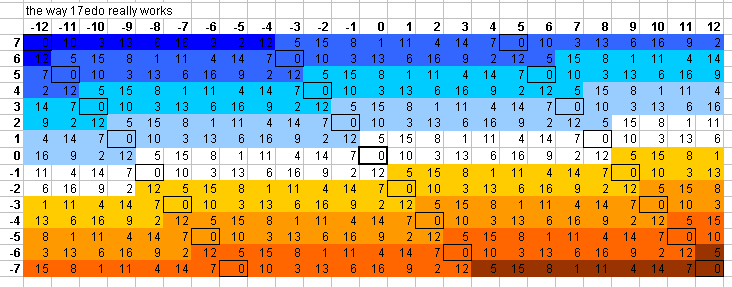
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 17edo:
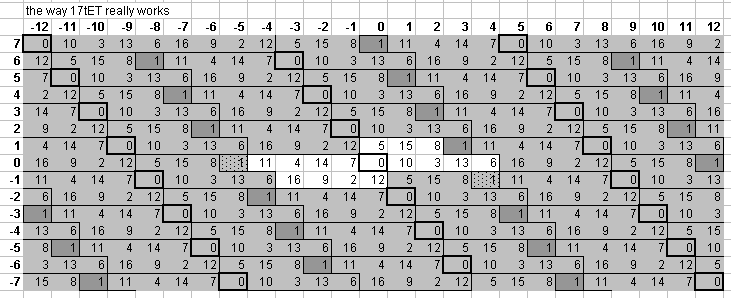
17edo tempers out the skhisma [8 1] and the 5-limit chromatic semitone [-1 2] (called "small semitone" by Ellis).
17edo does not temper out the syntonic comma, kleisma, diaschisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, semicomma, or ampersand's comma.
Because of the fact that the 5-limit "3rds" ( major and minor) are both represented by 5 degrees of 17edo, which is really a "neutral 3rd", it cannot emulate 5-limit tuning well, but rather functions primarily as a Pythagorean tuning, with 4 and 6 degrees being good representations of [-3 0] (i.e., 3-3) and [4 0] (= 34), respectively.
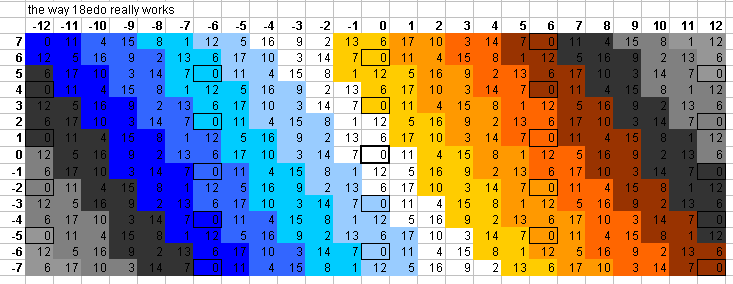
18edo
19edo
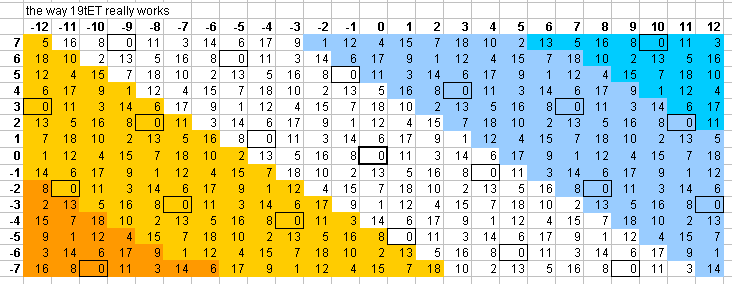
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 19edo:

19edo tempers out the syntonic comma [4 -1], causing it to act as 1/3-comma meantone; and also the kleisma [-5 6] and "magic" comma [-1 5], and others derived from those three.
19edo does not temper out the skhisma, diaschisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, semicomma, or ampersand's comma.
20edo

21edo

22edo
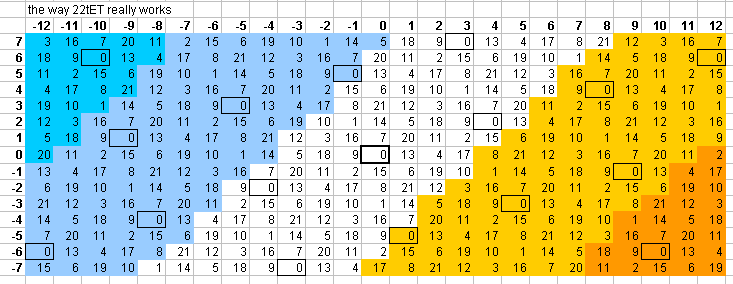
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 22edo:

22edo tempers out the diaschisma [-4 -2], the "porcupine" comma [-5 3], the "magic" comma [-1 5], the semicomma [3 7], and their derivatives.
22edo does not temper out the syntonic comma, skhisma, diesis, Pythagorean comma, or ampersand's comma.
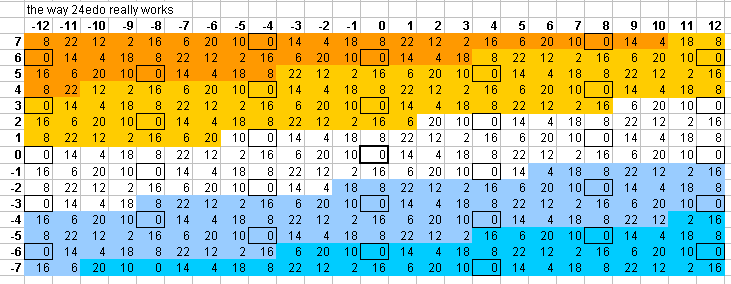
24edo
26edo
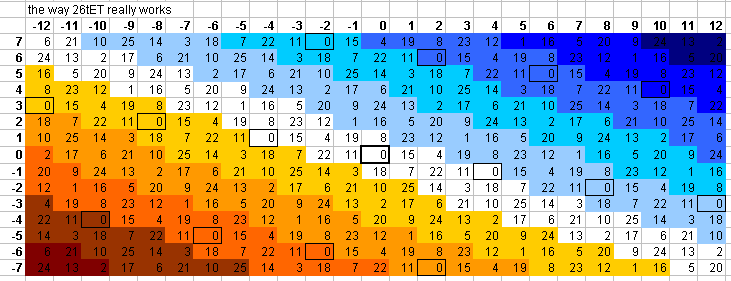
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 26edo:

26edo tempers out the syntonic comma [4 -1], causing it to act as 4/9-comma meantone.
27edo
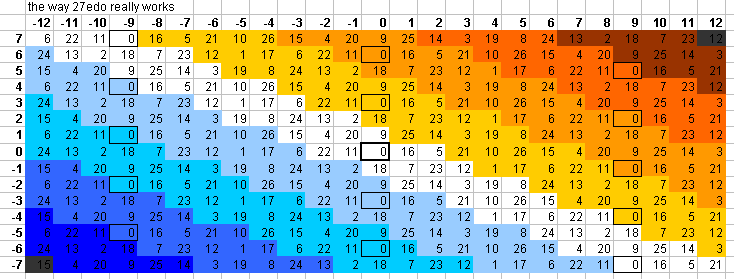
28edo
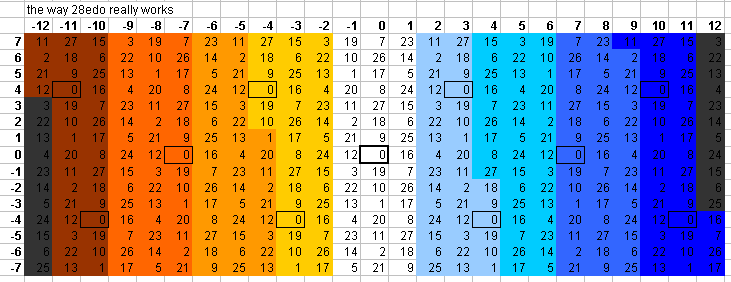
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 28edo:
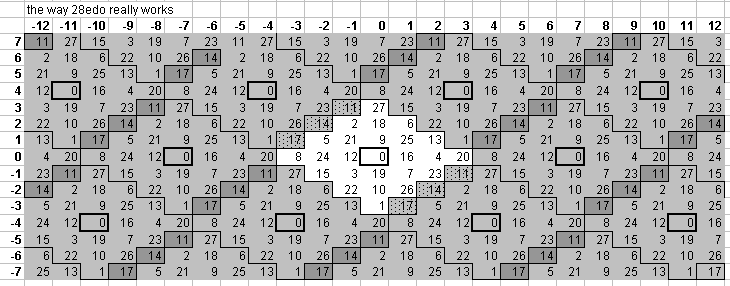
29edo
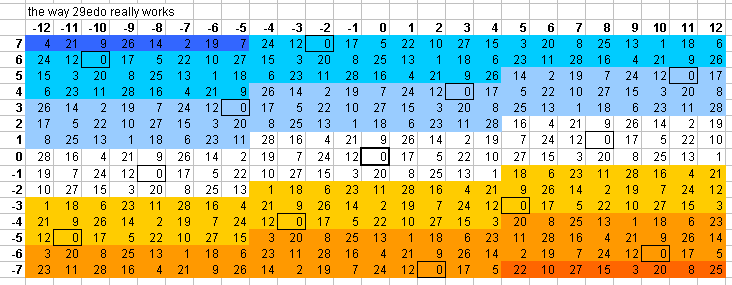
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 29edo:
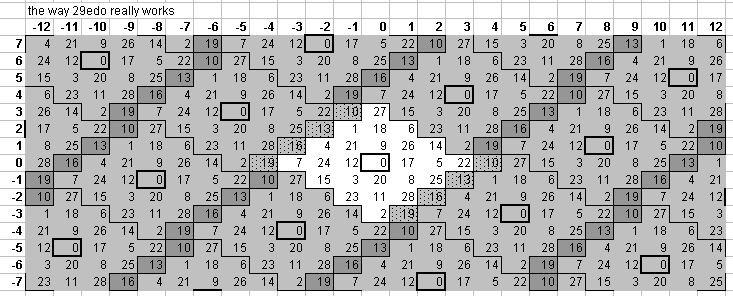
31edo

Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 31edo:

31edo tempers out primarily the syntonic comma [4 -1], which causes it to act as 1/4-comma meantone; and also the semicomma [3 7], ampersand's comma [7 6], and others derived from these three. The tempering-out of ampersand's comma means that 31edo also belongs to the MIRACLE family of temperaments.
31edo does not temper out the skhisma, diaschisma, diesis, Pythagorean comma, kleisma, minimal diesis, porcupine comma, or magic comma.
For an alternative view, below is a hexagonal bingo-card lattice of 31edo adapted from a graphic by Paul Erlich (mine are all rectangular). Hexagonal lattices give both of the basic 5-limit intervals, the 5/4 and the 6/5, as one step on the lattice. Here, the 23 intervals which are uniquely close to the 1/1 are shaded in green, and the 8 pairs which have two occurences equally far from 1/1 are shaded in bluish-grey.
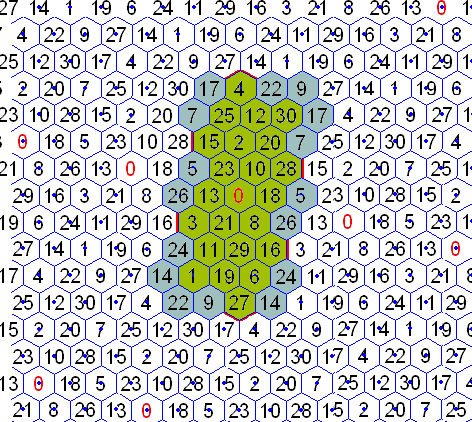
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 19-out-of-31edo scale which is a close approximation to one of the most common forms of meantone in Renaissance Europe (probably the closest thing to a "standard tuning" for instrumental music in this repertoire); the central periodicity-block is notated as letters and letters-with-accidentals:
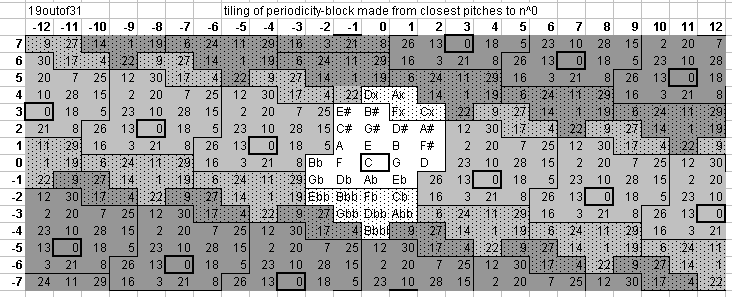
34edo
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 34edo:
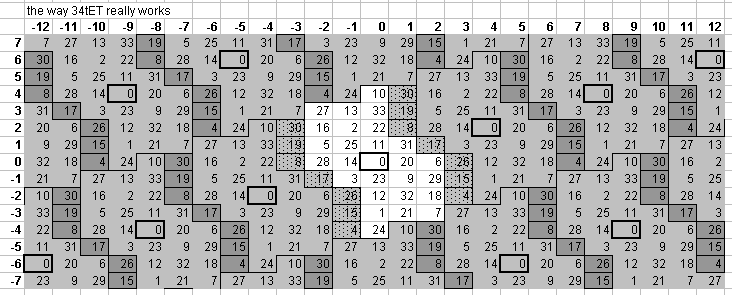
34edo tempers out the diaschisma.
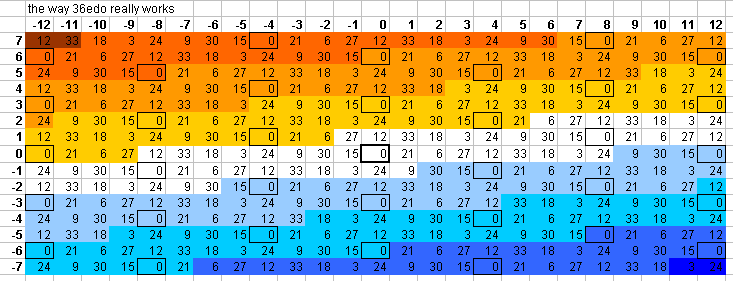
36edo
40edo

Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 40edo:
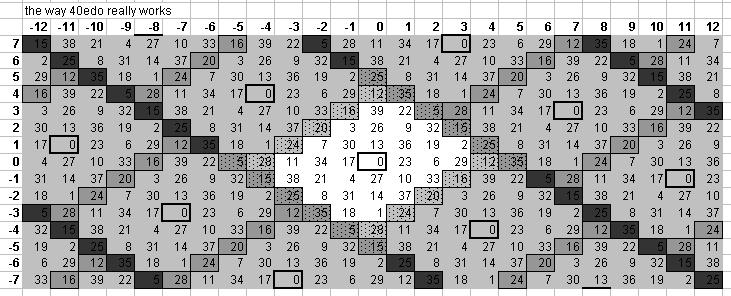
41edo
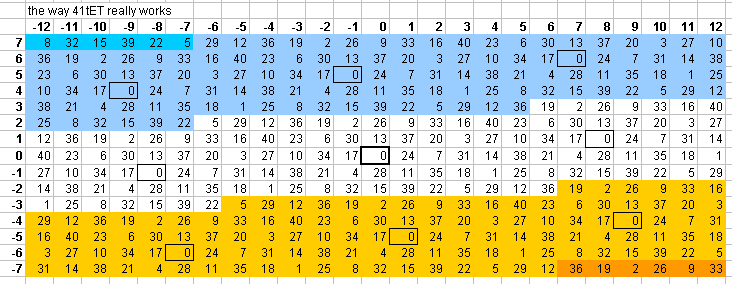
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 41edo:
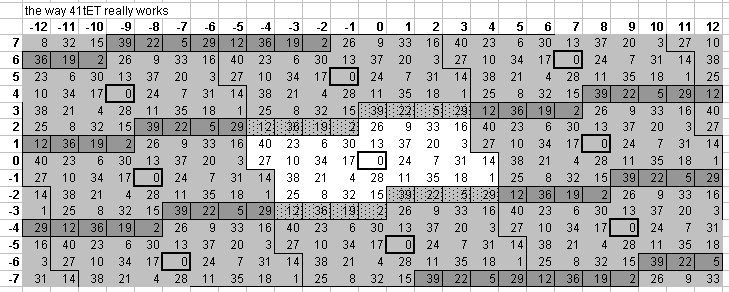
41edo tempers out the skhisma [8 1], minimal diesis [-9 4], magic comma [-1 5], ampersand's comma [7 6], and their derivatives. The tempering-out of ampersand's comma means that 41edo also belongs to the MIRACLE family of temperaments.
41edo does not temper out the syntonic comma, diaschisma, diesis, Pythagorean comma, kleisma, or semicomma.
43edo
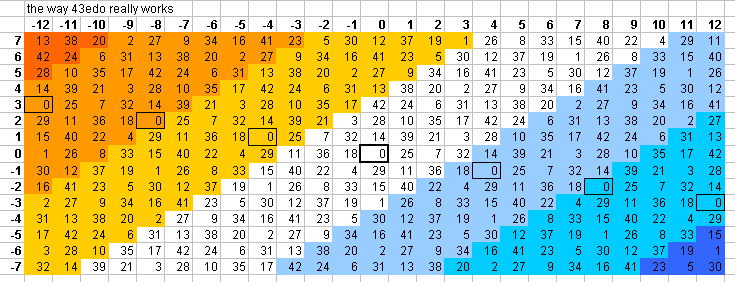
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 43edo:
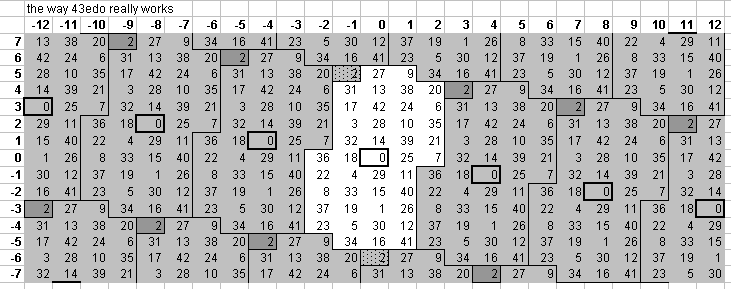
43edo tempers out the syntonic comma, causing it to act as 1/5-comma meantone.
43edo does not temper out the kleisma, skhisma, diaschisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, semicomma, ampersand's comma, or magic comma.
see also: meride
46edo
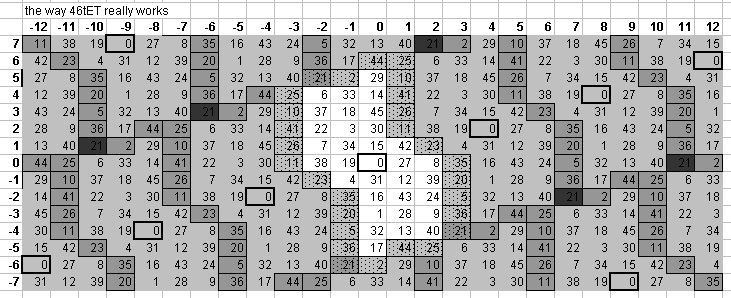
46edo tempers out the diaschisma and its multiples.
46edo does not temper out the syntonic comma, kleisma, skhisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, semicomma, or ampersand's comma.
46edo provides excellent approximations to basic intervals in not only in the 5-limit (as in this lattice), but also in 7- and 11-limit, as can be seen on the equal-temperament page.
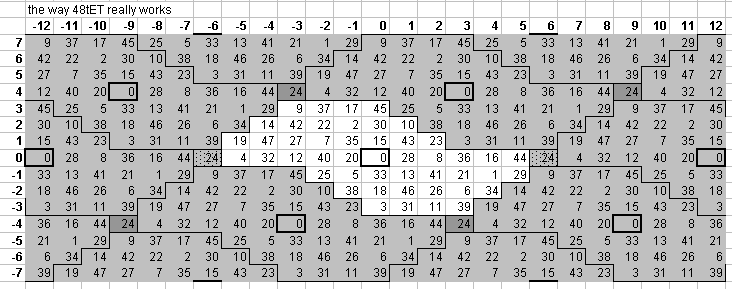
48edo
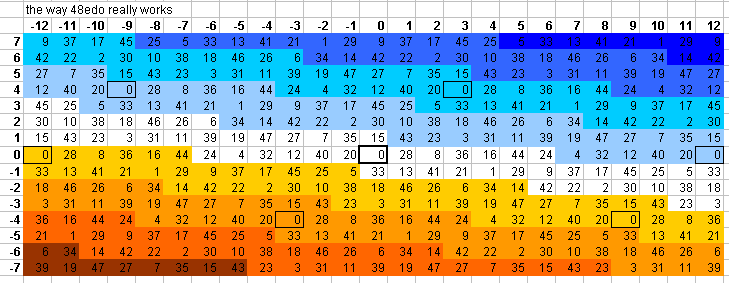
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 48edo:
50edo

50edo tempers out the syntonic comma [4 -1], causing it to act as 2/7-comma, 5/18-comma, and 7/26-comma meantone. It is also an excellent approximation of golden meantone. Along with its multiples, this is the only common small 5-limit interval tempered out by 50edo; another different one is [10 10], which has not yet been named.
50edo does not temper out the diaschisma, diesis, Pythagorean comma, kleisma, semicomma, minimal diesis, magic comma, or ampersand's comma.
50edo thus gives a good emulation of many JI harmonic structures, while at the same time avoiding the problem of commatic drift.
51edo
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 51edo:

51edo tempers out the porcupine comma [-5 3] and ampersand's comma [7 6]; thus, it is a member of the porcupine and MIRACLE families of temperaments.
51edo does not temper out the syntonic comma, kleisma, skhisma, diaschisma, diesis, Pythagorean comma, minimal diesis, magic comma, or semicomma.
52edo
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 52edo:
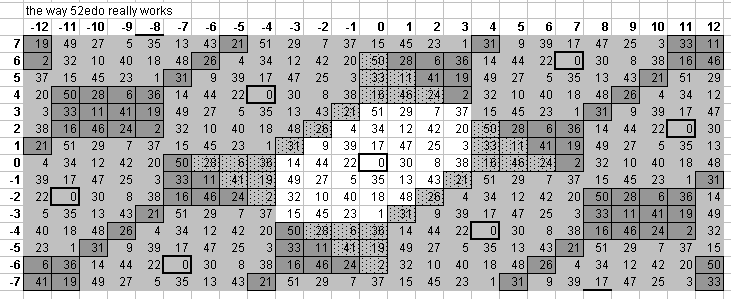
53edo
Note that for this big section of the lattice, there is no error between the 53edo bingo-card lattice and that showing the nearest 53edo approximation to JI. This means, therefore, that 53edo provides a superb system of integer interval-measurement for most 3-limit Pythagorean and 5-limit JI tunings.
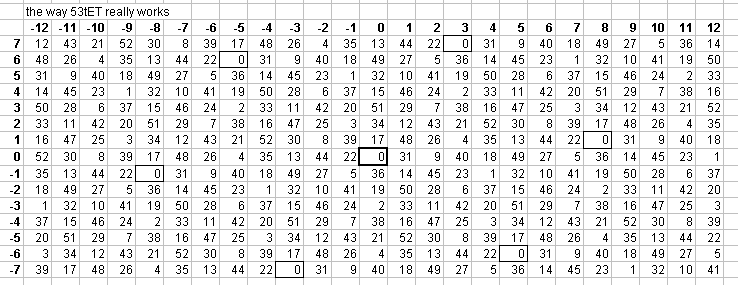
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 53edo:
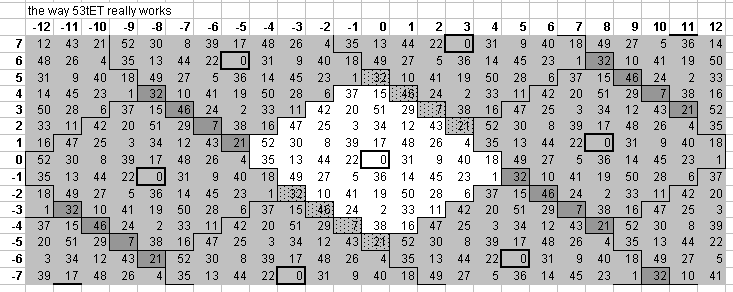
53edo tempers out the skhisma [8 1], kleisma [-5 6], and semicomma [3 7], and their derivatives.
53 edo does not temper out the syntonic comma, diaschisma, diesis, minimal diesis, magic comma, or ampersand's comma.
54edo
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 54edo:
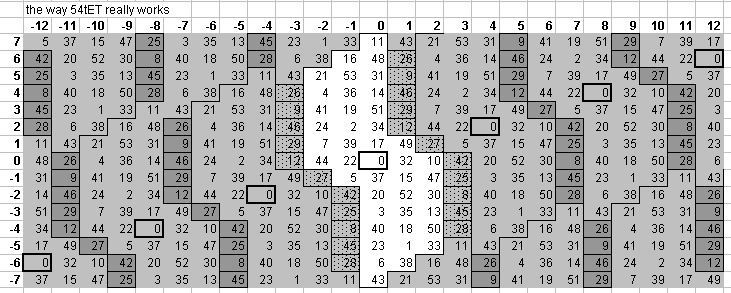
54edo tempers out the diaschisma [-4 -2] and its multiples.
54edo does not temper out the syntonic comma, kleisma, skhisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, magic comma, semicomma, or ampersand's comma.
55edo
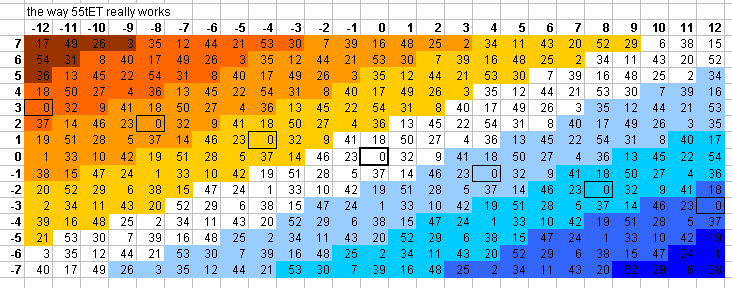
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 55edo:
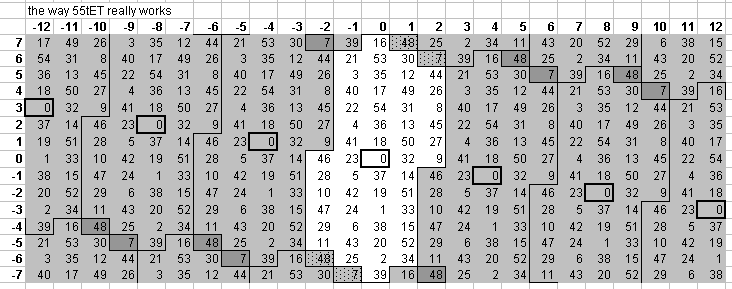
55edo tempers out the syntonic comma [4 -1], causing it to act as 1/6-comma meantone. The central periodicity-block is defined by the syntonic comma and the {3,5} interval-vector [-3 -13] = ~72.05672 cents. Two other commas which are tempered out by 55edo are [19 9] and [-15 -10], which have not yet been named.
55edo does not temper out the diaschisma, diesis, Pythagorean comma, kleisma, semicomma, minimal diesis, magic comma, or ampersand's comma.
55edo thus gives a good emulation of many JI harmonic structures, while at the same time avoiding the problem of commatic drift.
64edo
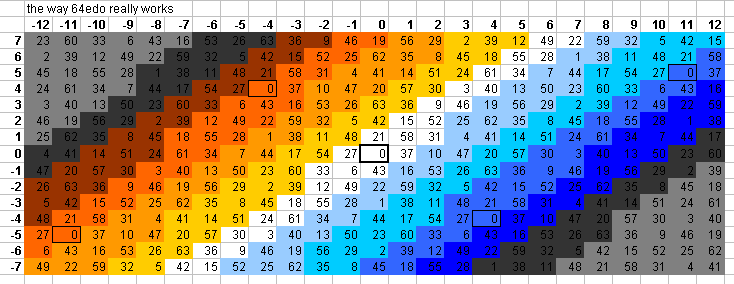
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 64edo:
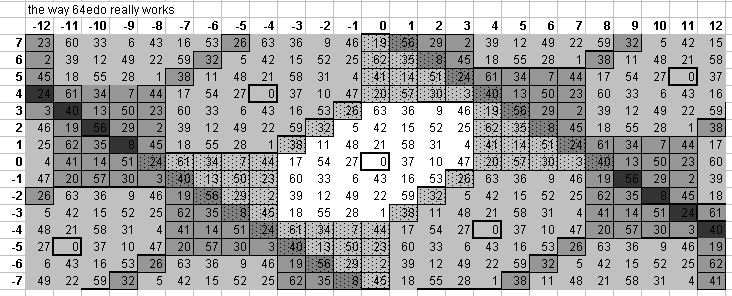
65edo
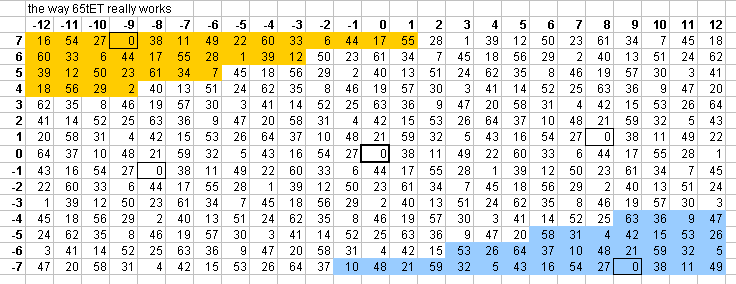
65edo tempers out the skhisma [8 1].
65edo does not temper out the syntonic comma, kleisma, diaschisma, diesis, Pythagorean comma, minimal diesis, porcupine comma, magic comma, semicomma, or ampersand's comma.
72edo
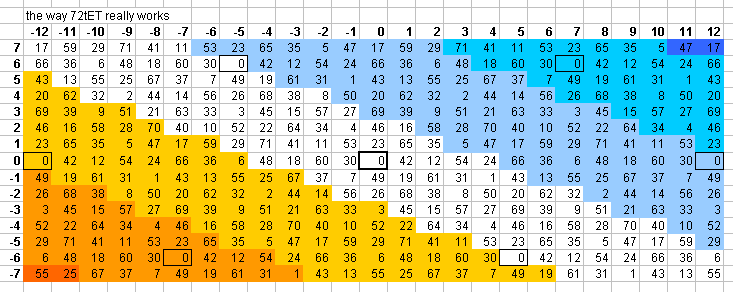
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 72edo:
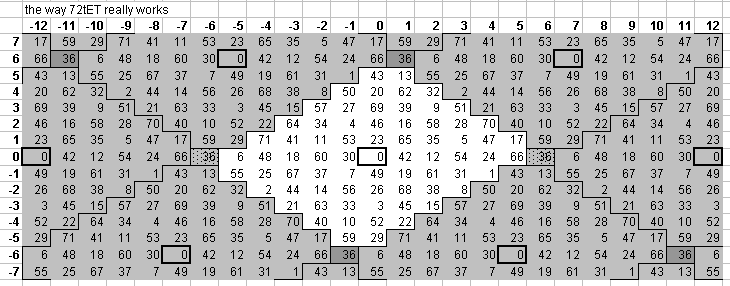
72edo tempers out the kleisma [-5 6], Pythagorean comma [12 0], and ampersand's comma [7 6], and their derivatives.
72edo does not temper out the syntonic comma, skhisma, diaschisma, diesis, minimal diesis, porcupine comma, magic comma, or semicomma.
The fact that 72edo's approximations do not temper out so many important 5-limit intervals means that, even tho it is not as accurate as the lower-cardinality 53edo in representing the entire lattice, its structure does emulate so many of the important JI commas that in a systemic sense it is easily perceived as a good approximation of JI.
118edo
Below is a lattice which shows one pattern by which the plane of the [3 5] lattice is tiled by 118edo:
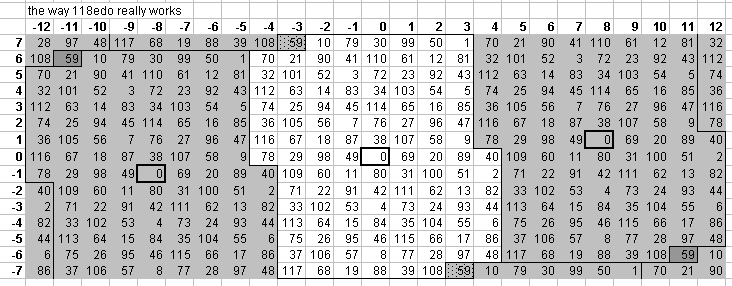
768edo
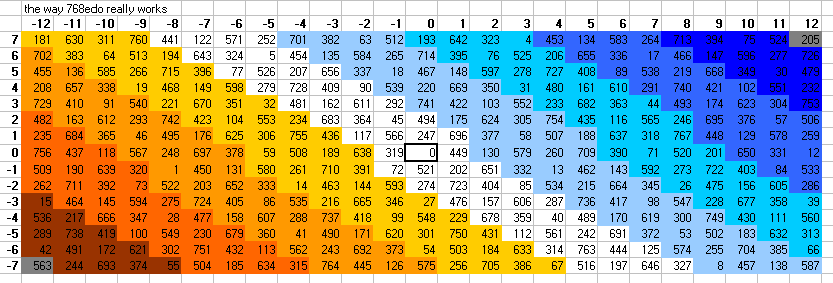
768edo is the default maximum tuning resolution of a large selection of MIDI hardware. This lattice shows the best mapping of 5-limit JI to 768edo, where 449 degrees == 3:2 and 247 degrees == 5:4. It is a fine enough resolution that all of the common "commas" are represented and none tempered out.
Comparison of different EDO bingo-card lattices
©2002 by Joe Monzo
Comparison of different EDO bingo-card lattices
©2002 by Joe Monzo
MouseOver the cardinality names of the various
EDOs
to see a bingo-card lattice of them showing the
error from the closest EDO approximation to
JI.
[NOTE: unfortunately, this applet is disabled on the Yahoo website]
MouseOver the cardinality names of the various
EDOs
to see a bingo-card lattice of them showing the
tiling of the central
periodicity-block at the
distance of other
unison-vectors.
[NOTE: unfortunately, this applet is disabled on the Yahoo website]
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32 33 34 35
36
37 38 39
40
41
42
43
44 45 46 47
48
49
50
51 52
53
54
55
56 57 58 59
60 61 62 63
64
65
66 67 68 69
70 71
72
73 74 75 76 77 78 79

10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32 33
34
35
36
37 38 39
40
41
42
43
44 45
46
47
48
49
50
51
52
53
54
55
56 57 58 59
60 61 62 63
64
65
66 67 68 69
70 71
72
73 74 75 76 77 78 79
118
Updated:
2002.10.22 -- added "tiling" lattices and explanation
2002.10.16 -- expanded explanation under "12edo"
2002.10.12 -- page created