An acronym I use to stand for Helmholtz / Ellis / Wolf / Monzo notation (the long name was first used by Paul Erlich on the Tuning List).
My version of the notational system uses prime-factors and their exponents as accidentals placed before the note-heads, along with the following accidental symbols, whose intonational inflections are given in the table:
prime-factorization
lower raise 2 3 5 7 11 ratio ~cents
b # [-11 7 0 0 0] 2187:2048 113.6850061
v ^ [ -5 1 0 0 1] 33:32 53.2729432
< > [ 6 -2 0 -1 0] 64:63 27.2640918
- + [ -4 4 -1 0 0] 81:80 21.5062896
These symbols for the accidentals were chosen specifically with the view towards being able to communicate about rational tunings by email using the standard ASCII character set. The mathematical basis up to the 5-limit, and the process of prime-factorization, is described more fully here. This exposition will focus on the accidental symbols.
If indication of prime-factor 2 is required, it may simply be added to the list of exponents in the accidental. Normally "8ve"-equivalence is assumed, so prime-factor 2 can simply be ignored, as it will be in the following discussion. In terms of the notation, this means that a letter-name, or any combination of a letter-name and accidentals, represents any "8ve" of that note. Thus, we are here dealing with pitch-classes as opposed to specific pitches.
(I use a vector notation here in which each vector element is an exponent of a prime-factor, the prime series implied in order from left to right, beginning with 3. For example,
[ 1 ] = 31 = 3 , [ 0 1] = 51 = 5 , [ 1 1] = 3151 = 15 , [-1 1] = 3-151 = 5/3,
etc. I use "redundant coding" here to illustrate all four types of nomenclature: letter-names, prime-factor vectors, ratios, and cents. The math works out correctly for all four: add letters alphabetically, add elements of vectors, multiply ratios, and add cents. Ratios are thus the most difficult to comprehend of the group, and cents the least accurate.)
The Pythagorean basis - handling of prime-factor 3
Essentially, I take the historical Pythagorean and diatonic origins of standard western musical notation as my starting point. The different powers (exponents) of prime-factor 3, create notes with different letter-names for the 7 notes of the diatonic scale:
letter F C G D A E B 3^x, x= -4 -3 -2 -1 0 1 2 ratio 128/81 32/27 16/9 4/3 1/1 3/2 9/8 ~cents 792 294 996 498 0 702 204
Their ordering here, by exponent of prime-factor 3, creates the beginning of the "circle (or cycle) of 5ths (or 4ths)". Placed in order of pitch, these 7 notes compose the Pythagorean diatonic scale:
letter A B C D E F G 3^x, x= 0 2 -3 -1 1 -4 -2 ratio 1/1 9/8 32/27 4/3 3/2 128/81 16/9 ~cents 0 204 294 498 702 792 996
Incidentally, it can be seen that the Pythagorean "diatonic semitone" is thus 3-5 or [-5] = 256:243 ratio = ~902/9 cents:
letter 3^x ratio ~cents
F [-4] 128:81 792
- E - [ 1] / 3:2 - 702
----- ------ -------- -----
= [-5] 256:243 90
C [-3] 32:27 294
- B - [ 2] / 9:8 - 204
----- ------ ------ -----
= [-5] 256:243 90
and that the Pythagorean "tone" is 32 = [2] = 9:8 ratio = ~20310/11 cents:
letter 3^x ratio ~cents
B [ 2] 9:8 204
- A - [ 0] / 1:1 - 0
----- ------ ------ -----
= [ 2] 9:8 204
D [-1] 4:3 498
- C - [-3] / 32:27 294
----- ------ ------ -----
= [ 2] 9:8 - 204 ... etc.
These two intervals are the step-sizes of the Pythagorean scale. This scale was assumed as the theoretical standard in European music for about 1000 years, from c. 500 to c. 1500 AD. The standard reference is Boethius, de institutione musica (books 1, 2, 3, 4, 5).
Going back to the "cycle of 5ths" presentation, adding a note to either end:
letter Bb F C G D A E B F# 3^x, x= -5 -4 -3 -2 -1 0 1 2 3 ratio 256/243 128/81 32/27 16/9 4/3 1/1 3/2 9/8 27/16 ~cents 90 792 294 996 498 0 702 204 906
requires the use of an accidental: b on the flat (negative) side and # on the sharp (positive) side. Ignoring prime-factor 2 since we assume "8ve"-equivalence, we may describe this as a change of "plus" or "minus" (which when using ratios means dividing or multiplying) 37 = 2187:2048 = ~1132/3 cents:
letter 3^x ratio ~cents F# [ 3] 27:16 906 - F - [-4] / 128:81 - 792 ----- ------ -------- ----- # = [ 7] 2187:2048 114 Bb [-5] 256:243 90 - B - [ 2] / 9:8 - 204 ----- ------ --------- ----- b = [-7] 2048:2187 -114
Essentially, # and b indicate chromatic alteration of diatonic scale tones within a Pythagorean (that is, 3-limit) context. They thus designate the Pythagorean "chromatic semitone" of ~1132/3 cents.
(Note that German nomenclature is quite unorthodox in including the 8th letter, H. See http://www.ixpres.com/interval/dict/german-h.htm for a detailed examination.)
Symbols for prime-factor 5
Examining the introduction of accidentals by increase in the prime series, the next set we find are + and -, which indicate the presence of prime-factor 5, and represent an intonational inflection of "plus" or "minus" 81:80 = [4 -1], the syntonic comma of ~211/2 cents.
| E- [-3 1] B- [-2 1] F#- [-1 1] C#- [0 1] G#- [1 1]
exponent 1 + 40:27 10:9 5:3 5:4 15:8
| 680 182 884 386 1088
|
| C [-3 0] G [-2 0] D [-1 0] A [0 0] E [1 0]
of 0 + 32:27 16:9 4:3 1:1 3:2
| 294 996 498 0 702
|
| Ab+ [-3 -1] Eb+ [-2 -1] Bb+ [-1 -1] F+ [0 -1] C+ [1 -1]
5 -1 + 256:135 64:45 16:15 8:5 6:5
| 1108 610 112 814 316
|
-----|-----+-------------+------------+-----------+----------+------
| -3 -2 -1 0 1
e x p o n e n t o f 3
So it can be seen, for example, that the just "major 3rd" (with ratio 5:4) above C [-3 0] , is E- [-3 1] , so therefore:
letter 3 5 ratio ~cents E- [-3 1] 40:27 680 - E - [ 1 0] / 3:2 - 702 ---- --------- ------- ----- - = [-4 1] 80:81 -22
And this C [-3 0] itself has a counterpart a comma higher at C+ [1 -1] :
letter 3 5 ratio ~cents C+ [ 1 -1] 6:5 316 - C - [-3 0] / 32:27 - 294 ---- --------- ------- ----- + = [ 4 -1] 81:80 22
As a parenthetical digression here, it is worth pointing out that the vast majority of music in the standard Euro-centric repertoire never distinguishes this interval, so that an A is always simply an A, a B is always simply a B, etc. This indicates that these composers always intended to temper out (i.e., make vanish) the syntonic comma.
The tuning systems most obviously implied by this are meantone, well-temperament, or an equal-temperament which has this feature, the most conspicuous being 12edo, and a comprehensive list including also 19, 24, 26, 31, 43, 45, 50, 55, 69, 74, 81, and 88edo.
The tempering-out of this interval also has the effect of broadening the meaning of # and b in these tunings, as follows:
[ 7 0] + [4 -1] = [11 -1] 177147 : 163840 135.1912957
[ 7 0] 2187 : 2048 113.6850061
[ 7 0] - [4 -1] = [ 3 1] 135 : 128 92.17871646
[ 3 1] - [4 -1] = [-1 2] 25 : 24 70.67242686
[-1 2] - [4 -1] = [-5 3] 250 : 243 49.16613727
[-5 3] - [4 -1] = [-9 4] 20000 : 19683 27.65984767 etc.
So these intervals are all functionally identical in these types of tunings, and so, depending on the harmonic context, # and b can "mean" an intonational inflection by any of these 5-limit intervals. So any of these intervals (and by extension on either side, possibly some others as well) may be implied as the "chromatic semitone" in music of this repertoire.
With this broadening of meaning for "chromatic semitone", there is a corresponding broadening of meaning for "diatonic semitone" as well:
ratio ~cents
[ 3 -2] + [4 -1] = [ 7 -3] 2187 : 2000 154.7438645
[-1 -1] + [4 -1] = [ 3 -2] 27 : 25 133.2375749
[-5 0] + [4 -1] = [-1 -1] 16 : 15 111.7312853
[-5 0] 256 : 243 90.22499567
[-5 0] - [4 -1] = [-9 1] 20480 : 19683 68.71870608 etc.
(See http://www.ixpres.com/interval/dict/no-syncom.htm for a detailed examination of this.)
So only a close analysis of the harmonic context of a given passage of music can disclose which of these are intended to be implied. Often, composers write chord progressions in which several of these ratios may be implied simultaneously, which blurs distinctions between them. Tuning theorists generally refer to this as "punning".
Using HEWM notation, a composer would not be able to create puns based on the syntonic comma, because the comma is indicated explicitly in the notation. The composer would have to find other intervals which are not designated in this notation, to choose for punning.
Now, back to the main narrative ...
Symbols for prime-factor 7
The next prime in the series is 7, and so the next set of accidentals we find are > and <, which represent the presence of prime-factor 7, by indicating an intonational inflection of "plus" or "minus" 64:63, as follows:
Referring to the scale above, the 7th harmonic (or partial) of our reference pitch A [0 0 0] would be notated as G< [0 0 1], to indicate that it is ~271/4 cents lower (flatter) than the G [-2 0 0] we have already obtained in our basic scale, thus:
letter 3 5 7 ratio ~cents G< [ 0 0 1] 7:4 969 - G - [-2 0 0] / 16:9 - 996 ---- ------------ ------ ----- < = [ 2 0 1] 63:64 -27
letter 3 5 7 ratio ~cents B> [ 0 0 -1] 8:7 231 - B - [ 2 0 0] / 9:8 - 204 ---- ------------ ------ ----- > = [-2 0 -1] 64:63 27
Note that meantone tunings provide an interval which is close to the 7th harmonic, namely the "augmented 6th" of +10 generators in the meantone "cycle of 5ths". Thus, for example, a meantone A# == HEWM Bb<. The use of "augmented 6th chords" by European "common-practice" composers thus seems to indicate a liking for the 4:5:6:7 sonority, as the proportion of the "augmented 6th chord" in meantone tuning very nearly approaches 4:5:6:7.
Symbols for prime-factor 11
The last set of new accidentals in my HEWM notation indicate the presence of prime-factor 11, by indicating an intonational inflection of "plus" or "minus" 33:32, as follows:
Referring again to the basic Pythagorean scale, the 11th harmonic (or partial) of our reference pitch A [0 0 0 0] would be notated as D^ [0 0 0 1], to indicate that it is ~531/4 cents higher (sharper) than the D [-1 0 0 0] we have already obtained in our basic scale, thus:
letter 3 5 7 11 ratio ~cents D^ [ 0 0 0 1] 11:8 551 - D - [-1 0 0 0] / 4:3 - 498 ---- --------------- ------ ----- ^ = [ 1 0 0 1] 33:32 53
letter 3 5 7 11 ratio ~cents Ev [ 0 0 0 -1] 16:11 649 - E - [ 1 0 0 0] / 3:2 - 702 ---- ------------ ------- ----- v = [-1 0 0 -1] 32:33 -53
I've made an ExcelTM spreadsheet available at http://sonic-arts.org/dict/hewm.xls. Using prime-factor vector format, the user can enter a target ratio and a succession of either approximate prime-factor vectors of ratios or HEWM notational symbols, in order to finally arrive at the HEWM notation of that ratio.
And here's another spreadsheet which allows the user to convert pitch-classes from a large subset of Ben Johnston's notational system into HEWM: http://sonic-arts.org/monzo/johnston/johnston-hewm.xls.
The tempered version: "simplified HEWM" based on 72edo
The rational basis of my HEWM notation translates
very well into my 72edo notation:
lower raise inflection cents b # semitone 100 v ^ 1/4-tone 50 < > 1/6-tone 331/3 - + 1/12-tone 162/3
The 72edo version of HEWM simplifies things a great deal, because of the fact that multiples of certain symbols may equal other symbols, and thus sets of symbols which equal opposites cancel each other out and may be omitted from the notation.
Here's a great example of how the JI version of HEWM differs from the 72edo version:
Let's say we want to find the notation for the ratio 42:25, assuming that C = 1/1.
The first thing to do on the spreadsheet is to make sure that there's a zero next to "C" in the column of cyan cells near the top. This defines "C" as 1/1.
Then put in the target ratio. Whether using the method
of inputting "comma" exponents or inputting the notation
directly, the result will be the same:
2 3 5 7 11
target ratio: [ 1 1 -2 1 0] 42/25 898.2
HEWM: B bb - [ 15 -9 0 0 0] 32768/19683 882.4
-------------------
[-14 10 -2 1 0] 413343/409600 15.7
< - [ -6 2 0 1 0] 63/64 -27.3
-------------------
[ -8 8 -2 0 0] 6561/6400 43.0
+ - [ -4 4 -1 0 0] 81/80 21.5
-------------------
[ -4 4 -1 0 0] 81/80 21.5
+ - [ -4 4 -1 0 0] 81/80 21.5
-------------------
[ 0 0 0 0 0] 1/1 0.0
So the JI HEWM notation for 42:25 is Bbb<++.
This is simplified in 72edo HEWM, because in 72edo, < = -- and > = ++, so we have Bbb<>, and the <> cancel each other out, leaving us with just Bbb.
In the JI version of HEWM, > and ++ are not the same, and so therefore all the symbols must be employed for precision.
Daniel Wolf's version of HEWM
The references at the end of this paper also mentions Daniel Wolf's version of HEWM, which is essentially identical to mine except for the use of different symbols for the prime-factors, and Wolf covers all primes up to 23:
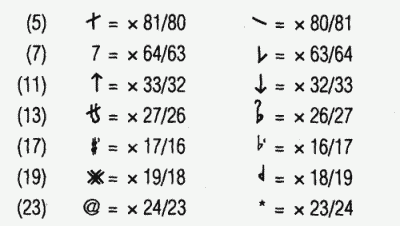
prime-factorization
2 3 5 7 11 13 17 19 23 ratio ~cents
[-11 7 0 0 0 0 0 0 0] 2187:2048 113.6850061 (symbol: the Pythagorean # and b)
[ -4 4 -1 0 0 0 0 0 0] 81:80 21.5062896
[ 6 -2 0 -1 0 0 0 0 0] 64:63 27.2640918
[ -5 1 0 0 1 0 0 0 0] 33:32 53.2729432
[ -1 3 0 0 0 -1 0 0 0] 27:26 65.33734083
[ -4 0 0 0 0 0 1 0 0] 17:16 104.9554095
[ -1 -2 0 0 0 0 0 1 0] 19:18 93.6030144
[ 3 1 0 0 0 0 0 0 -1] 24:23 73.6806536
Note that Wolf's symbols for prime-factor 5, 7, and 11 are very similar to mine. Also, note that he cleverly alludes to # and b for both prime-factors 17 and 19, whose inflections are both larger than the others and in the vicinity of a semitone.
In private communication, Mr. Wolf sent me the following update on his noational ideas:
. . .
I believe that we are in substantial agreement about notation. I like the historical approach, assuming octave equivalence, retaining the staff and using the series of fifths with seven nominals and two accidentals to represent a pythagorean sequence. Further, because I am a vocally oriented composer (and most of the instruments I play -- early winds, javanese rebab -- are voice-like in their pitch orientation), hearing from interval to interval, and tending to compose in a locally tonal but globally less-tonal way, I need to have invariant interval sizes: when I see a perfect fifth on the staff, without any modifications, I want to imagine a perfect fifth and not have to go through extraordinary mental gymnastics in order to figure out that it should actually be a comma-shy or comma-too-big).
This is not to ignore the fact that such pythagorean sequences can be mapped to an indefinite number of linear temperaments. In doing so, it may be useful to establish conventions of equivalence such as that found in meantone, where four ascending fifths are octave-equivalent to a just third (or in a skhismatic temperament where eight descending fifths do the same job).
When we wish to use tunings using intervals other than those found in a pythagorean tuning (or tempered equivalent thereof), then I advocate the use of additional accidental, indicating "comma" shifts from the pythagorean values. "Comma" is taken here broadly to indicate simply small intervals, with my preference to intervals with powers of two or three on one side of the ratio. The accidentals should come in clear pairs, either logical pairs (plus/minus) or graphic inversions.
In _1/1_ I proposed a series of such accidentals. I stand by the symbols printed there through the 11-limit and have used them successfully with performers for over twenty years. The rest were thought up in haste in the days of daisy wheel printers and the limitations of a fixed set of characters; consequently, I do not stand by the particular graphics but the principles respresented by the signs remain valid. I will here propose some improvements to the graphics, but with the caveat that I have not yet found it neccessary to use such a notation, as my own music in JI has either been restricted to an 11-limit, or has gone so far beyond such a limit as to require a notation with some combination of ratios, exact frequency, and/or cent deviations from 12tet..
For shifts of 81/80 and 80/81, the syntonic comma, I use plus and minus signs respectively. This follows Erv Wilson, from whom I have also taken the practice of slanting significantly broadened horizontal strokes to ca. 45 degrees for increased readability and distinction from the staff lines. The horizontal stroke on the plus sign slants upward, that on the minus sign downward, the direction of the slant intended to increase the sense of direction conveyed by the interval. (I find that building a bit of redundancy into a notation is not a significant violation of my need for elegance in a notation).
For shifts of 63/64 and 64/63, the septimal comma, indicating ratios involving a factor 7, I use a numeral seven and its inversion. In ASCII, one can either use a "7" and a capital letter "L", but the greater-than and less-than signs (>,<) are pretty good substitutes. This seems to be an uncontroversial accidental, although those who prefer to notate the septimal minor seventh as an augmented sixth might well have a different opinion. (In 31tet, you can have it both ways: an augmented sixth has the same size as a minor seventh diminished by the interval equivalent in that temperament to the septimal comma, in this case one step of 31tet).
With the introduction of ratios involving a factor 11, I had, initially, to decide upon an orientation. The tone found 11/8 above a given tonic is almost exactly a quartertone between the perfect fourth and the augmented fourth above a given tone. Consequently, I could notate the 11/8 as either a fourth with an accidental raising by the interval 33/32 or as an augmented fourth diminished by the interval 729/704. I went with the simpler ratio and the added bonus that I would probably encounter fewer compound accidentals (i.e. . I notate this accidental with up and down arrows, following a common convention for notating quartertones.
At this point, I should note that the graphics selected for the the syntonic and unadecimal commas may be confused with those used in one of the current 72tet notations (there are several competing systems out there, none of which can be considered standard; I suppose that the recent advocacy of 72 by conductor and composer Hans Zender might push things in his direction, but I'll do a wait and see on this!) . In some of these systems, arrows are used for 12th tone deviations, which are very close in size to a syntonic comma, and a modified square root sign is used for the quartertone accidental. In contrast, both the Ben Johnston system for JI and the one described here use pluses and minuses for the syntonic comma -- we just disagree about the content of the set of pitches _without_ accidentals. I could cop out and simply say that my interest is in notating extended Just Intonations and the 72tet-ers can do there own thing, but the affinity of 72 for creating a near-just environment is not to be undervalued, and the notation described here functions identically to the Sims system when used in 72tet. It is equally efficient and just differs in the choice of graphics.
However, I'm willing to offer support for my choice of graphics, and one piece of explicit criticism for the Sims set. When I wrote my item for 1/1, I was writing my dissertation and supporting myself by teaching Junior-year Algebra in a Catholic Girl's Priory School in Southern California. At the time, I was convinced about the septimal accidentals, but wasn't sure whether pluses/minus were to be assigned to syntonic commas and up/down arrows to the 11-limit commas or vice versa. Erv Wilson had prepared me to like pluses and minuses at the smaller comma, pluses and minus were used in some of the important 19th century theory to indicate syntonic commas, and I had seem some quartertone scores with arrows, but that was hardly conclusive. So I made a totally unscientific poll on the subject and asked 124 Catholic Girl's Priory School 11th graders their opinion on the question:
"In your opinion, which of the following signs better indicates a larger quantity: (a) an arrow pointed upward (by means of a graphic of same drawn in white chalk on a green board) or (b) a plus sign (with this graphic drawn by similar means)?
The answer to this wholly unscientific poll of was clear: 102 chose (a), the upward-pointing arrow, 20 chose (b), the plus sign, and the remaining two were apparently agnostic.
So I went with the scheme described above.
And oh yes, my explicit criticism of the Sims: the modified square root signs are too large, and I find them, personally, to be ugly.
As mentioned before, the signs in the _1/1_ article (by the way, although the editors of the journal call it "one-one" when pronouncing the name of their journal, following the pronunciation favored by Partch and Harrison for musical ratios, there are minority schools of practice where the name is pronounced as either "one to one" (which is somewhat sporting), "one over one" (which is too focused on power for my taste) or "one on one"; but then, maybe the name is not in English in the forst place: the German _eins zu eins_ shares the sporting connotation, while _eins durch eins_ or _eins über eins_ both have an historical-political aftertaste; the Hungarian _egy egy_, on the other hand, sounds enough like fingernails scraping against a clean, dry chalkboard to convince me that _Magyar_ was not a pronounciation choice foreseen by said editors, but I digress: enough of that!) were made hastily, under primitive technological circumstances and are herefore, hereby _und hiermit_ retracted immediately and irrovocably.
For ratios involving the factor 13, I propose to notate the intervals 26/27 and 27/26 with a question mark and an inverted question mark. The association of things tredecimal with strangness is not unknown (just ask anyone of us who happen to have been born on the 13th of September) and a question mark suggests this quality well. In the _1/1_ item, I tried, unhappily, to combine question marks with sharps and flats; I now find the question marks to be adequate on their own. Fortunately, Spanish punctuation requires the inverted question mark
Ratios involving factors of 17 and 19 represent very small deviations from the accidentals found in the original pythagorean set. Again, as in the unadecimal accidental, one has an orientation issue with which to deal, but in this case there is no advantage vis a vis compounding accidentals, but I can go with the simplest ratios. In _1/1_, I proposed adding a comma or apostrophe attached to a sharp, flat or natural for 17/16 and 16/17. This was, in itself, not objectionable for the sharp-sighted, but the signs for ratios of 19 (513/512 and 512/513) were just plain silly. I propose instead to keep sharp, flats, and naturals and simply add 17 or 19 above or below the accidental to indicate modifications by the relevant ratio in the direction of the numeral relative to the pythagorean accidental. For example, a c proceeded by a sharp with a seventeen above it would represented the frequency of C multiplied by 17/16. (You could also think of it as C# (2187/2048) multiplied by 2176/2187, but the simpler ratios seem more useful to me). This notation can be extended arbitrarily upward to ratios based on higher prime numbers.
There is more to be discussed -- for example, the problems of notating pitches related by several factors. At a certain point, the signage does get unwieldy (that point was rapidly reached in trying to notate a hebdomekontany!) and one might as well go with some combination of explicit ratios, frequencies, and cent-deviations from 12tet. Also, I have to admit that I have also developed a number of notational shorthands that I constantly use while composing but have hesistated to spring on performers. The shorthand I use use most frequently is a composite flat and septimal lowering, where the top, horizontal, line of the numeral seven extends from the vertical shaft of the flat. I further admit to being fond of the look of my shorthand, but this falls squarely into the box of personal eccentricities, is part of my sketching hand, not my clean copy, and is not intended or suggested for wider use.
Dr. Daniel James Wolf
Composer, Budapest/Morro Bay
http://home.snafu.de/djwolf/
Manuel Op de Coul's version of HEWM (used in Scala)
prime-factorization
lower raise 2 3 5 7 11 ratio ~cents
b # [-11 7 0 0 0] 2187:2048 113.6850061
v ^ [ -5 1 0 0 1] 33:32 53.2729432
L 7 [ 6 -2 0 -1 0] 64:63 27.2640918
\ / [ -4 4 -1 0 0] 81:80 21.5062896
The Hauptmann / Helmholtz / Ellis / Eitz versions of HEWM
The version of HEWM described by Hermann Helmholtz and Alexander Ellis is in Ellis's 1875 English translation of Helmholtz's On The Sensations of Tone.
As Paul Erlich noted, "apparently the HEWM notation system traces it roots to Eitz in 1891 and still further back to Hauptmann". Dave Benson, course notes for Mathematics and Music (zip file), Chapter 5, p 116 and 117, gives an explanation of Eitz's notation:
Eitz devised a system of notation, used in Barbour, which is convenient for describing scales based around the octave. His method is to start with the Pythagorean definitions of the notes and then put a superscript describing how many commas to adjust by. Each comma multiplies the frequency by a factor of 81/80.As an example, the Pythagorean E, notated E0 in this system, is 81:64 of C, while E-1 is decreased by a factor of 81/80 from this value, to give the just ration of 80:64 or 5:4.
In this notation, the basic scale for just intonation is given by
C0 - D0 - E-1 - F0 - G0 - A-1 - B-1 - C0 .
A common variant of this notation is to use subscripts rather than superscripts, so that the just major third in the key of C is E-1 instead of E-1.
Benson's footnote about Eitz:
Carl A. Eitz, Das mathematisch-reine Tonsystem, Leipzig, 1891. A similar notation was used earlier by Hauptmann and modified by Helmholtz.
Daniel Wolf also suggest that I point out that prolific German theorist Martin Vogel also uses + and - for the syntonic comma.
[from Joe Monzo, JustMusic: A New Harmony]
updated: 2002.3.9, 2002.3.5, 2002.2.23, 2002.2.8