An examination of Fox-Strangways and Partch on JI modulation
webpage and soundfiles © 2000 by Joe Monzo
Citation from Partch's book (p 191-192)
Harry Partch. [1949] 1974. Genesis of a Music.
2nd edition: Da Capo Press, New York.
xxv + 517 pages.
Paperback republication 1979, ISBN 0-306-80106-X pbk.
Fox-Strangways on the Subject
A few years ago a letter from A. H. Fox-Strangways, founder and
first editor of the English quarterly Music and Letters,
came to me through an intermediary. Fox-Strangways has been
an active critic and a forceful intellectual participant in
every recent movement or significant discussion looking
toward the expansion of musical resources, and the letter
I hold is very excellent from several standpoints. It
embraces, succinctly and clearly, a good many of the beliefs
and prejudices of the average well-educated musician in
regard to modulation, melodic subtleties in
harmonic music,
and any tampering with
Equal Temperament
theory. Not every
creative theorist has his opposition directed into a nutshell,
and since the points made by Fox-Strangways must be answered,
it is obvoiusly desirable to reproduce his letter virtually
in full8. The letter was in answer to an article on
Monophony
sent to Fox-Strangways through the aforesaid
intermediary. This highly chivalrous message, and gracious,
considering that it betokened a rejected manuscript,
follows:1
June 8, 1934.
Dear Sir:
I'm afraid I can make no use of this, for the following reason.
There are many ways of securing
just intonation
- it depends on
(1) what accuracy (2) what practicability you want. This seems
to be a good way as far as I understand it.
But the unfortunate thing is that we don't want just intonation:
it would stop off the simplest modulation - e.g.,
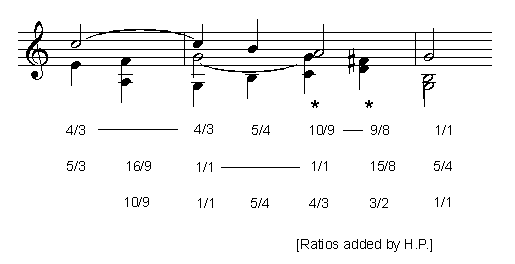
In the chords
* * , A is taken as 80/48 [10/9] and left as
81/48 [9/8] of C. If you have two A's to represent this
then there is no modulation - i.e., the power
of taking a note in two senses.
To go back from Equal Temperament on keyed instruments is to
scrap the music of two centuries. We may have entered on
an evil course - it has ruined singing, for instance - but
we shall have to go on with it - probably in Yasser's direction
[see page 3042 -Partch],
though I can't at present see how
the difficulties are to be got over. What your man [= Partch]
says about Indian and Arab scales is true, but irrelevant:
they were for melody, and we wnat harmony. The Indians
are "up against it," too: they have imported the harmonium,
the issue of which is inevitably European harmony, though
they don't know it. A 25-note harmonium [it is actually
23 tones to the 2/1 - see page 2683
-Partch] has been invented for them,
so that they can play their rags, but they won't use it - too
difficult, too expensive - they are settling down
complacently on the 12-note scale, and contenting themselves with the
dozen or so rags it will play, and scrapping the
many scores of them they used to sing.
A few of them hope for a great Preservation Trust? but the
old rags are rapidly disappearing as Sanskrit is -
too difficult, and we're too busy. It's all very sad.
Again, music is not made by acoustic specialists but by
the musical geniuses, who - Elgar, for instance - "don't
know what the supertonic is" and persist in "taking
their music from the air," i.e., from what they manage to
think out of any rotten old fiddle or trombone
they may happen to possess4:
and they accept any kind
of temperament that gives scope for modulation,
which is vital to thinking. Your man
will probably say he can modulate. So he can; but
at an expense of "grey matter" that the ordinary
practitioner is not going to contemplate....
There - I've no time, though there's lots more "to it" - sorry.
Yours very truly,
(Signed) A. H. FOX-STRANGWAYS.
Getting Down to Cases
The accuracy of Just Intonation has not proved "practicable" for a single reason: the lack of significant music for instruments conceived for Just Intonation and incapable of anything else. In Equal Temperament we have achieved a fine balance between a scale as painless as possible5 and one which can produce the Well-Tempered Clavichord.6 Equal Temperament has proved "practicable" simply because the music written for its instruments is significant.
The modulation in question has been played on the Chromelodeon7 for many musicians, including a graduate class at the Eastman School of Music in Rochester, New York, and at a lecture at the University of Wisconsin, in three ways: sustaining the 10/9 through the third and fourth beats, sustaining the 9/8 through the third and fourth beats, playing the 10/9 on the third beat and 9/8 on the fourth (which is necessary if both chords are in just tuning). My own reaction is that the difference in quality of the resolving chords is obvious, and that this has little or no bearing on the achievement of the modulation, which is successful in all three instances. The three types were not announced to my hearers, and no one suggested that there was no modulation in any case.9 The great capacity of Mnophony for taking a tone in two or more senses has been amply discussed (see Chapter 8). 8
Notes by Partch:
8 Permission kindly granted by Fox-Strangways in a message dated June 6, 1945.
9 After the playing of this modulation at the University of Wisconsin one man correctlhy stated - from the audience - the nature of the chords in the three renditions. This man was an amateur violinist who had heard neither me nor my music before. Several others told me afterward that they had also correctly guessed the modulations. I also asked the specific question: "Was the modulation accomplished? If not, in which playing was it not accomplished?" No one was at all sure that the modulation had not been accomplished in all three instances. I afterwards explained, of course, that any hesitation whatever invalidates the theory that Just Intonation "stops off" modulation.
Notes by Monzo:
1 I find more than a hint of sarcasm in Partch's response here.
2 A misprint, probably inadvertently carried over from the 1st edition. The correct page number for the 2nd edition is p 431.
3 Another misprint as above; the correct page is p 395.
4 This brings to mind Beethoven's great comment to his violinist Schuppanzigh. When the latter complained of the difficulty of a new piece, Beethoven's retort was: 'Do you believe I think about your whining little fiddle when the Great Creator speaks to me?!'.
5 It is interesting that in 1999, John deLaubenfels created an adaptive JI algorithm in which the primary goal was to reduce what he termed the 'pain' of the deviation from 'ideal tuning'.
6 This piece by Bach is now generally known in English as the Well-Tempered Klavier. The 'Clavichord' is an English name for a specific instrument, whereas 'Klavier', the German term used by Bach, is a generic word which can refer to any member from the entire family of keyboard instruments. It is has also now been established that Bach's intended tuning for this piece was not 12-tET, as Partch consistently assumed in his attacks, but rather one of the well-temperaments that was in common use in Bach's time, as Bach's title clearly indicates. There is a significant difference, in that the pitches in a well-temperament are not equally-spaced, so that each key has its own characteristic set of intervals and therefore a unique sound, which is not the case in 12-tET. The assumption that the piece was intended to illustrate equal-temperament was an error that was propogated in the musico-historical literature for about 200 years, until quite recently, and Partch was merely citing what was in his time common, but incorrect, knowledge.
7 The Chromelodeon was a harmonium (reed organ) adapted by Partch to play his microtonal scale. He made several different versions. In my audio examples, I used the General-MIDI 'reed organ' patch; it has the advantage in listening to tuning illustrations that the sound is sustained and without vibrato; it has a fairly rich harmonic timbre.
8 Pages 119-137 in the 2nd ed.
Further commentary by Monzo
Partch presented only three examples in his demonstration, and always resolved back to the 1/1-Otonality. While this was sufficient to refute the specific points made by Fox-Strangways, his experiment was thus somewhat biased, by omission, in favor of his own theories.
He did not include in his demonstration any examples of the modulation which could illustrate further the basis of Fox-Strangways's criticism, where the common-tones are held over at the same frequency and the structure of the chords remain exactly the same as they would be in Partch's third case (i.e., where 'both chords are in just tuning').
This would result in some sort of commatic drift, which is the problem that forms the basis of Fox-Strangways's major argument. Partch proved his own point but left this question unexamined. Let's take a look.
To begin with, the first of the chords marked with a star in Fox-Strangways's example is rather curious: because of the particular set of pitches in this chord, there is no possible way, within the 5-limit we're assuming here, to get all the proportions in a very-low-integer relationship, as in all the other chords in the example. Because of the existence of both 'G' and 'A', there must be a 9 as a factor in this chord, either in an 8:9 ratio or in a 9:10. Fox-Strangways could have used a chord here which could be analyzed into 4:5:6 proportions (in other words, a plain 'subdominant' triad for this chord), and still have made his point.
The chord is functioning as an abbreviated 'subdominant major 6th chord', without the '3rd': C-G-A. While the normal expectation would be to give the interval C:G in just-intonation as a 3:2 'perfect 5th', the 'A', the 'major 6th', can be given as either the 5:3 or 27:16 ratios. 5:3 relates it to the 'subdominant', while 27:16 relates it to the 'dominant'.
Alternatively, the chord can also be analyzed as a 'first inversion supertonic 7th', written 'ii6/5' (these last numbers are not a frequency ratio; they should be written stacked one over the other), with the '5th' omitted. The ii chord is where the problem of the commatic shift arises, but it could have been illustrated with a simple I-vi-ii-V progression in one key, without a modulation.
So apparently, he deliberately chose to use as his 'pivot chord' a set of pitches that had some ambiguity in its structure.
In all these illustrations, the lattice diagrams are drawn using the 'triangular' convention (as usually done on the Tuning List), for each chord. The two triangles which outline the 4/3- and 1/1-Otonalities (the two key-centers in question in this modulation) are always shown as a reference, even where some or all notes are missing from that chord. The first four chords are the same in all examples. We'll make a thorough examination of Partch's three examples first.
Partch's 'instance 1' gives the first starred chord the 'just' proportion 6:9:10, sustains the 10/9 as the '5th' of the dominant chord (the second starred chord) against the expected 'root' and 'major 3rd' of this chord, so that this dominant chord has the highly dissonant proportion 108:135:160 (= 4 : 5 : ~5.93 , 4:5:6 = 108:135:162), and then resolves to the expected 1/1-Otonality. While the 15/8 'F#' in the second starred chord forms a 5:4 'major 3rd' with the 3/2 'G' which is the 'root', the 10/9 'A' is a comma flat, and with 3/2 it forms the dissonant '5th' having the ratio 40:27, a comma narrower than the 3:2 'perfect 5th'.
| 4/3
5/3 ---
5:3 ----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 10/9
1/1 4/3
10:9----- -----
A\ / \ / \
\ / \ / \
\ / \ / \
4:3-----1:1------
C G
| 10/9
15/8 3/2
10:9----- ----- -----15:8
A\ / \ / \ /F#
\ / \ / \ /
\ / \ / \ /
----- -----3:2
D
| 1/1
5/4 1/1
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
|
Partch's 'instance 2' gives the first starred chord the fairly dissonant proportion 16:24:27, sustains the 9/8 so that the second starred chord is 'in just tuning', with the proportion 4:5:6 as the expected 'dominant', and resolves onto the expected 'new tonic', the 1/1-Otonality.
| 4/3
5/3 ---
5:3-----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 9/8
1/1 4/3
----- ----- -----
/ \ / \ / \ /
/ \ / \ / \ /
/ \ / \ / \ /
4:3-----1:1----- -----9:8
C G A
| 9/8
15/8 3/2
----- -----15:8
/ \ / \ /F#
/ \ / \ / \
/ \ / \ / \
----- -----3:2----9:8
D A
| 1/1
5/4 1/1
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
|
Partch's 'instance 3' gives both of the starred chords 'in just tuning', the first with the proportion 6:9:10, and the second with the proportion 4:5:6, and resolves onto the expected 'new tonic', the 1/1-Otonality. As Partch stated, in order to give both chords 'in just tuning', the top voice has to give the 'A' at first as 10:9, then shift it up a comma to 9:8 to be consonant in the second chord.
| 4/3
5/3 ---
5:3-----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 10/9
1/1 4/3
10:9----- -----
A\ / \ / \
\ / \ / \
\ / \ / \
4:3-----1:1------
C G
| 9/8
15/8 3/2
----- -----15:8
/ \ / \ /F#
/ \ / \ / \
/ \ / \ / \
----- -----3:2----9:8
D A
| 1/1
5/4 1/1
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
|
Now we move on to some additional examples, still within the 5-limit, which Partch did not choose to illustrate. In many ways these are even more interesting.
In my first example, The first starred chord is given in Partch's 'just' version, with the proportion 6:9:10, then sustaining the 10/9 into a 4:5:6 on the second starred chord, making it a comma lower than the expected 'dominant', resolving finally onto a new 'tonic' which is a comma lower than the expected 1/1. This drift is precisely the problem that was so onerous for Fox-Strangways. To my ears, there is nothing wrong with the flattened 'dominant' chord, with only the final flattened 'tonic' sounding unusual. (...which prompted my next experiment...)
| 4/3
5/3 ---
5:3-----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 10/9
1/1 4/3
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
-----4:3-----1:1-----
C G
| 10/9
50/27 40/27
50:27
/F#
/ \
/ \
40:27----10:9---- -----
D A\ / \ / \
\ / \ / \
\ / \ / \
----- -----
| 160/81
100/81 160/81
100:81
/B\
/ \
/ \
160:81--- ----- ----- -----
G / \ / \ / \ / \
/ \ / \ / \ / \
/ \ / \ / \ / \
----- ----- ----- -----
|
Surprisingly enough, my second example doesn't sound the least bit objectionable to me. As in the example above, the first starred chord has proportion 6:9:10, and the 10/9 sustains into a 4:5:6 for the second starred chord, making it a comma lower than the expected 'dominant'. But then there is an abrupt shift up a comma, to resolve onto the new 'tonic' at the expected 1/1:
| 4/3
5/3 ---
5:3
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 10/9
1/1 4/3
10:9
A\ / \ / \
\ / \ / \
\ / \ / \
4:3-----1:1-----
C G
| 10/9
50/27 40/27
50:27
/F#
/ \
/ \
40:27----10:9---- -----
D A\ / \ / \
\ / \ / \
\ / \ / \
----- -----
| 1/1
5/4 1/1
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
|
Next we will sustain the 9/8 thru both starred chords. My third example keeps the C:G 3/2 '5th', but moves both of those pitches up a comma so that together with 9/8 they form Partch's 'just' proportion 6:9:10. This progresses to the expected 4:5:6 'dominant' chord which is a 3/2-Otonality. To my ears, the 'just' tuning of the pivot chord still sounds out-of-tune because of the comma-shifted pitches. (The example following this one will alter one note, to much better effect...)
| 4/3
5/3 ---
5:3-----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 9/8
81/80 27/20
-----
/ \ / \
/ \ / \
/ \ / \
----- ----- -----9:8-----
\ /A\ / \
\ / \ / \
\ / \ / \
----27:20---81:80
C G
| 9/8
15/8 3/2
15:8
/ \ / \ /F#
/ \ / \ / \
/ \ / \ / \
----- -----3:2-----9:8
D A
| 1/1
5/4 1/1
5:4
/ \ /B\
/ \ / \
/ \ / \
----1:1-----
G
|
In my fourth example, the first starred chord keeps the 9:8 proportion between the 'G' and 'A', and moves the 'C' up a comma from where it's expected so that it forms a consonant 6:5 'minor 3rd' with 'A'. The resulting dissonance with 'G' is one that I find not altogether unpleasant; see my further comments below. As above, it progresses to the expected 4:5:6 'dominant' chord which is a 3/2-Otonality.
| 4/3
5/3 ---
5:3-----
/E\ / \
/ \ / \
/ \ / \
4:3---- ------
C
| 4/3
16/9 10/9
10:9----- -----
/A\ / \ / \
/ \ / \ / \
/ \ / \ / \
16:9----4:3----- -----
F C
| 4/3
1/1 1/1
-----
/ \ / \
/ \ / \
/ \ / \
4:3----1:1-----
C G
| 5/4
1/1 5/4
-----5:4
/ \ /B\
/ \ / \
/ \ / \
-----1:1-----
G
| 9/8
1/1 27/20
-----
/ \ / \
/ \ / \
/ \ / \
-----1:1----- -----9:8-----
G \ /A\ /
\ / \ /
\ / \ /
----27:20
C
| 9/8
15/8 3/2
15:8
/ \ / \ /F#
/ \ / \ / \
/ \ / \ / \
----- -----3:2-----9:8
D A
| 1/1
5/4 1/1
5:4
/ \ /B\
/ \ / \
/ \ / \
----1:1-----
G
|
I think it's worth noting that, at least to my ears, musicians playing non-fixed-pitched instruments without accompaniment by fixed-pitched instruments (such as a capella singing, or string-quartet playing), often sound like they do such things as we hear in this last example. This is an area that definitely merits further study.
Finally, the last example is tuned in ordinary 12-tET.
A final note: Partch could have, and did (and so do many other JI composers) use many other different ratios to represent the intervallic gestalts that can be analyzed in this type of 'typical cadential progression'.
The examples here have all been within the 5-limit, but Partch's own arbitrarily chosen limit on his musical resources was the 11-odd-limit, so he had many other ratios with factors of 7, 9, and 11 at his disposal, in addition to all those that I have examined here, which were also within his system.
Most likely, Partch simply did not feel that it was necessary to complicate this particular examination more than necessary. It is clear from the musical example provided by Fox-Strangways that he was thinking of just-intonation in 'traditional' 5-limit terms. From what he wrote in his letter to Partch, it seems to me that he probably didn't actually explore Partch's system in much depth.
This brings up one last point I'd like to comment on. Partch ends his public response to Fox-Strangways with [2nd ed., p 193]: 'I am a bit nonplussed at the accusation of a relative absence of "grey matter" in my scale...'.
I've always felt that Partch misunderstood Fox-Strangways where the latter said that Partch could modulate 'but at an expense of "grey matter" that the ordinary practitioner is not going to contemplate'.
By my reading, it seems that, far from accusing Partch of 'a relative absence of "grey matter"' in his scale, Fox-Strangways is rather saying quite the opposite: that the ordinary musician would have to exert too much mental power to utilize Partch's system.
Tuning List commentary
The first example, John de Laubenfels COFT-1, is in one form of adaptive JI. It was calculated by John deLaubenfels using his algorithm, which gave the following results:
| note | cents
deviation
from 12-tET |
| B | -0.49 |
| A | -4.45 |
| G | +13.35 |
| F# | -20.27 |
| F | +9.39 |
| E | -2.45 |
| D | -6.43 |
| C | +11.37 |
Because all vertical sonorities here are already in JI, this is a COFT, which is a static adaptive JI.
Paul Erlich pointed out that if 'G' is taken as the 1/1, this is exactly the same as my second example above. How interesting that I intuitively chose as the best solution the same tuning produced by John deLaubenfels's algorithm...
Paul suggested that John sustain the 'D' into the final chord in his calculations. The MIDI-files I made continue to use the notes as in the original examples, without actually sounding that final 'D', even tho it is included in the algorithm.
This example is John de Laubenfels dynamic adaptive JI, weak vertical springs. (values indicate cents deviation from 12-tET):
| C +9.32
E -4.52 -- | C +9.34
F +8.01 A -4.92 | C +8.77
G +8.85 G +8.85 | B -6.62
G +8.40 B -6.62 | A -2.89
G +9.34 C +10.01 | A -1.26
F# -14.78 D +2.30 | G +5.66
B -8.50 G +5.66 (D +5.29) |
This example is John de Laubenfels COFT-2, derived from the above:
| note | cents
deviation
from 12-tET |
| B | -7.64 |
| A | -2.83 |
| G | +7.27 |
| F# | -14.79 |
| F | +8.01 |
| E | -4.52 |
| D | +4.31 |
| C | +9.32 |
This example is John de Laubenfels dynamic adaptive JI, strong vertical springs.
| C +10.90
E -2.94 -- | C +10.85
F +8.90 A -4.94 | C +9.96
G +11.94 G +11.94 | B -2.47
G +11.34 B -2.47 | A -3.02
G +14.76 C +12.78 | A +1.46
F# -14.36 D -0.49 | G +5.19
B -8.63 G +5.19 (D +7.17) |
Carl Lumma suggested this version which uses JI vertical sonorities shifted up or down slightly to eliminate drift. (Values are cents deviation from the ratios.):
| C 4/3
E 5/3 -- +/- 0.0 | C 4/3
F 16/9 A 10/9 +3.1 | C 4/3
G 1/1 G 1/1 +6.1 | B 5/4
G 1/1 B 5/4 +9.2 | A 10/9
G 1/1 C 4/3 +12.3 | A 9/8
F# 15/8 D 3/2 -6.1 | G 1/1
B 5/4 G 1/1 (D 3/2) -3.1 |
updates:
-
2000.08.31
2000.09.10
2000.09.11
