If we look at the brat ratios of Wendell Well, they are approximately [r, 3/2, 3/2, r, 3/2, 3/2, 3/2, r, 3/2, q, r, r] where both r and q are nearly 2, but r is a little less and q is a little more. If we solve for these under the condition that the product of the fifths comes to 128 (octave closure) then q is
320*(-27*r+18*r^2+40)/r/(2187*r^4-5184*r^3-7728*r+5760+5216*r^2)
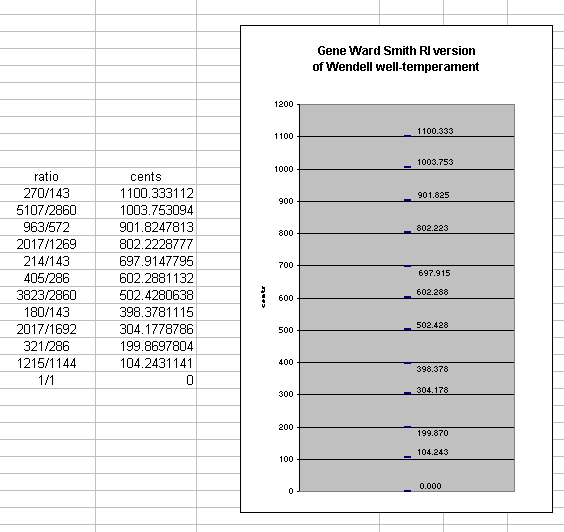
We can relate r and q in various ways; for instance making q exactly 2, or having the average of r and q be 2. The simplest way however is to make r exactly 2, which makes q to be 580/293. This gives us the following scale:
[1, 1215/1144, 321/286, 68187/57200, 180/143, 3823/2860, 405/286, 214/143, 22729/14300, 963/572, 5107/2860, 270/143]
It is a rational intonation version of Robert's temperament.
The tonalsoft.com website is almost entirely the work of one person: me, Joe Monzo. Please reward me for my knowledge and effort by choosing your preferred level of financial support. Thank you.