@ 00 A B C D E F G H I J K L M N O P Q R S T U V W X Y Z

Login   |  Encyclopedia Index

orwell

A name coined by Gene Ward Smith for the family of temperaments in which the semicomma vanishes (i.e., is tempered out).

The name is in honor of George Orwell, author of the book 1984, because the generator of one 11-limit version of this temperament (the 22&31 "george" variety) is 19/84-edo (AKA 19deg84).

5 limit

= semicomma

name:	orwell, semicomma
comma:	2,3,5-monzo |-21 3 7>
mapping:	[<1 0 3|, <0 7 -3|]
poptimal generator:	43/190-edo
TOP period:	1200.0 cents
TOP generator:	271.599422 cents
MOS cardinalities:	9, 13, 22, 31, 53, 84

Below are some Tonescape^® lattices of 5-limit orwell temperament.

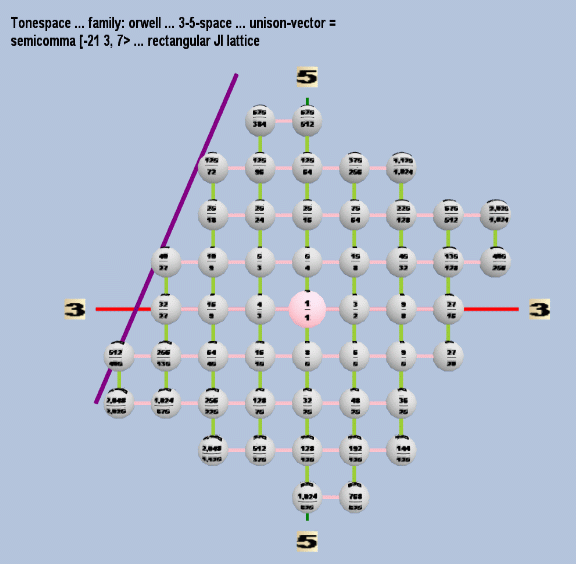
5-limit orwell, rectangular JI lattice:

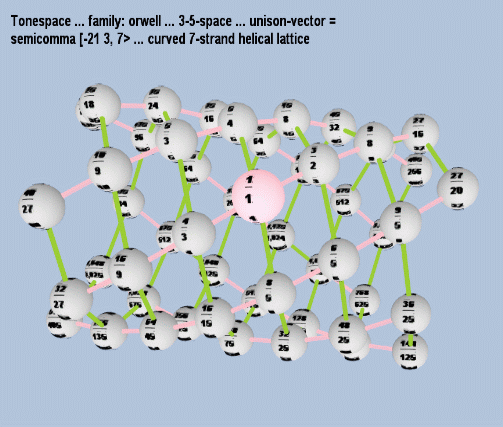
5-limit orwell, curved 7-strand helical lattice:

7 limit

name:	orwell, 22&31
wedgie:	<<7 -3 8 -21 -7 27||
mapping:	[<1 0 3 1|, <0 7 -3 8|]
7 limit poptimal generator:	26/115-edo
9 limit poptimal generator:	43/190-edo
other generators:	5/22-, 7/31-, 12/53-, 17/75-, 19/84-edo
TOP period:	1199.532657 cents
TOP generator:	271.493647 cents
TM basis:	{225/224, 1728/1715} ratios
MOS cardinalities:	9, 13, 22, 31, 53, 84

11-limit

name:	george, 22&31
wedgie:	<<7 -3 8 2 -21 -7 -21 27 15 -22||
mapping:	[<1 0 3 1 3|, <0 7 -3 8 2|]
poptimal generator:	19/84-edo
TOP period:	1201.251092 cents
TOP generator:	271.425083 cents
TM basis:	{99/98, 121/120, 176/175}
MOS cardinalities:	9, 13, 22, 31, 53, 84

name:	orwell, 31&84
wedgie:	<<7 -3 8 33 -21 -7 28 27 87 65||
mapping:	[<1 0 3 1 -4|, <0 7 -3 8 33|]
poptimal generator:	85/376-edo
TOP period:	1200.564417 cents
TOP generator:	271.443068 cents
TM basis:	{225/224, 441/440, 1728/1715}
MOS cardinalities:	9, 13, 22, 31, 53, 84, 115, 146

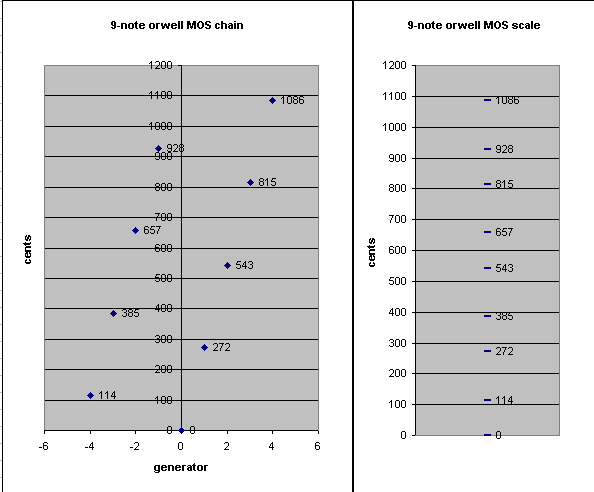
9-note MOS:

generator note  ratio

   -4     Eb+  (16/15)
   -3     F#-   (5/4)
   -2     A-<  (35/24)
   -1     B>   (12/7)
    0     D     (1/1)
   +1     F<    (7/6)
   +2     G+>  (48/35)
   +3     Bb+   (8/5)
   +4     C#-  (15/8)
		

Below are two graphs illustrating the example 9-note MOS given above: