Private lessons available in person in San Diego, or online using Discord: composition, music-theory, tuning-theory, piano, and all woodwinds (sax, clarinet, flute, bassoon, recorder). Current rates US$ 80 per hour (negotiable). Send an email to: monzojoe2 (AT) gmail. Also please consider making a donation to Tonalsoft using the PayPal button at the bottom of this page. Thank you.
The fretting of the Arab lute
© 1998, 2004 by Joseph L. Monzo
The lute became the most popular instrument in Europe
during the 1500s, similar to the guitar's popularity today.
The European instrument was derived from the Arab 'ud
("al-'ud" --> "lute"),
whose first flowering occurred during the 600s and 700s
in Syria and Iraq.
It is worth remembering thru-out this discussion that lute frets were always made of catgut and tied around the neck of the instrument, and could thus be moved at will, unlike the embedded metal frets on today's fretted string instruments.
The Arab lute's open strings and ancient fretting were
entirely Pythagorean
(that is, 3-limit), derived most
likely from
Sumerian/Babylonian,
Indian, and
Greek precedents.
The ancient tuning formed a 13-tone Pythagorean chain 3(-6 ... +6). This gave a scale which is audibly nearly the same as our modern standard 12edo tuning, but with two varieties of tritone.
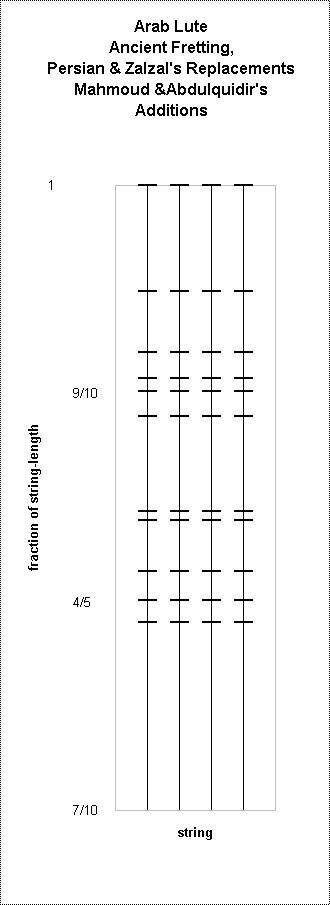
The ancient Arab lute fretting:
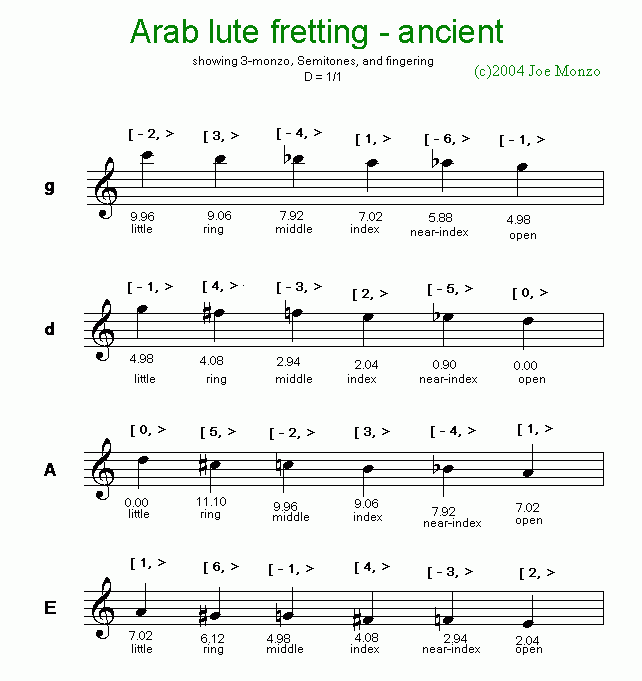
2,3,5,7,11-monzo ---------- ratio --------- string & finger string-length Semitones Ellis's cents [ 5 -2, 0 0 0 > 32 / 9 3.555555556 4 little 3 / 4 9.96 792 [ -3 3, 0 0 0 > 27 / 8 3.375 4 ring 64 / 81 9.06 702 [ 8 -4, 0 0 0 > 256 / 81 3.160493827 4 middle (ancient) 27 / 32 7.92 588 [ 0 1, 0 0 0 > 3 / 1 3.0 4 index 8 / 9 7.02 498 [ 11 -6, 0 0 0 > 2048 / 729 2.809327846 4 near-index (ancient) 243 / 256 5.88 384 [ 3 -1, 0 0 0 > 8 / 3 2.666666667 4 open = 3 little 1 / 1 4.98 294 [ -5 4, 0 0 0 > 81 / 32 2.53125 3 ring 64 / 81 4.08 204 [ 6 -3, 0 0 0 > 64 / 27 2.37037037 3 middle (ancient) 27 / 32 2.94 90 [ -2 2, 0 0 0 > 9 / 4 2.25 3 index 8 / 9 2.04 0 [ 9 -5, 0 0 0 > 512 / 243 2.106995885 3 near-index (ancient) 243 / 256 0.90 1086 [ 1 0, 0 0 0 > 2 / 1 2.0 3 open = 2 little 1 / 1 0.00 996 [ -7 5, 0 0 0 > 243 / 128 1.8984375 2 ring 64 / 81 11.10 906 [ 4 -2, 0 0 0 > 16 / 9 1.777777778 2 middle (ancient) 27 / 32 9.96 792 [ -4 3, 0 0 0 > 27 / 16 1.6875 2 index 8 / 9 9.06 702 [ 7 -4, 0 0 0 > 128 / 81 1.580246914 2 near-index (ancient) 243 / 256 7.92 588 [ -1 1, 0 0 0 > 3 / 2 1.5 2 open = 1 little 1 / 1 7.02 498 [ -9 6, 0 0 0 > 729 / 512 1.423828125 1 ring 64 / 81 6.12 408 [ 2 -1, 0 0 0 > 4 / 3 1.333333333 1 middle (ancient) 27 / 32 4.98 294 [ -6 4, 0 0 0 > 81 / 64 1.265625 1 index 8 / 9 4.08 204 [ 5 -3, 0 0 0 > 32 / 27 1.185185185 1 near-index (ancient) 243 / 256 2.94 90 [ -3 2, 0 0 0 > 9 / 8 1.125 1 open 1 / 1 2.04 0
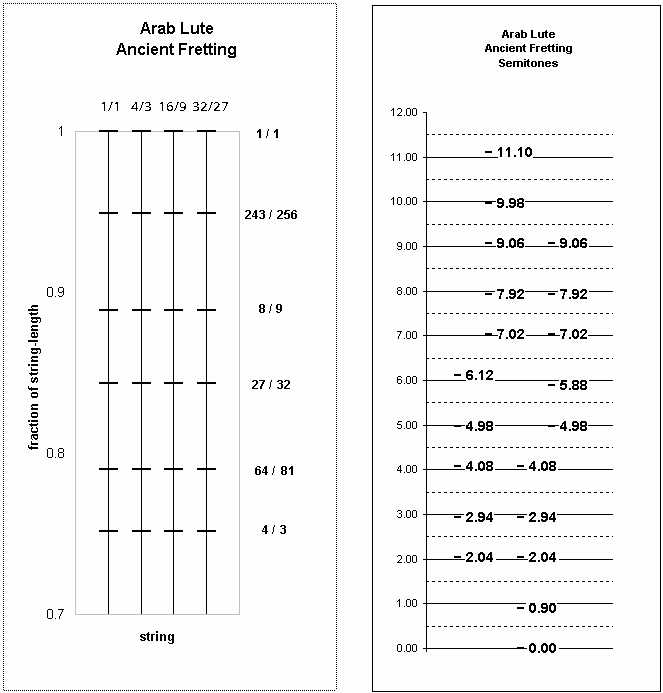
(Note that regular Pythagorean notation is sufficient for the ancient fretting.)
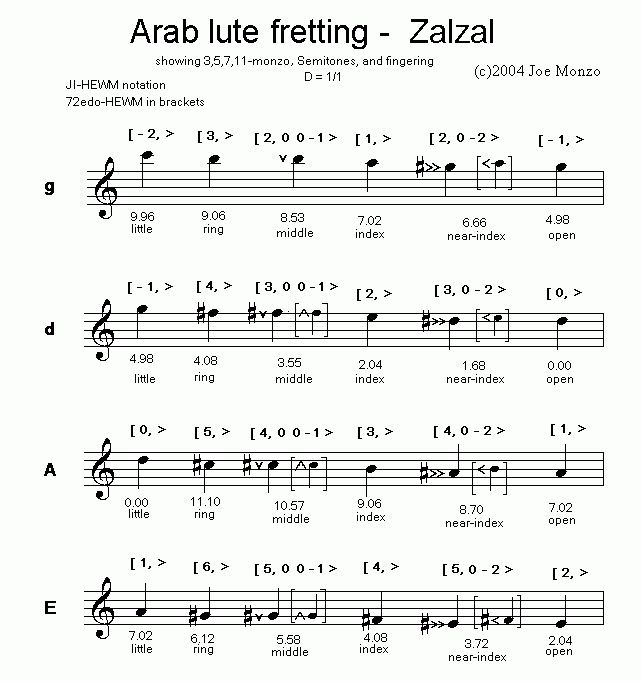
During the 8th century AD, Zalzal (d. 791) specified new higher
fret-placements for the "near-index" and "middle-finger" frets,
using respectively ratios of 7
and of 11. His refrettings resulted from equidistant string-length divisions.
Zalzal's 22/27 "middle-finger" fret results from a division of the string-length between 8/9 (the whole-tone) and 2/3 (the perfect-5th) into 3 equal parts: 8/9 = 24/27, 1/3 of the string distance above that = 22/27, and 2/3 of the string distance above that = 18/27 = 2/3. As the lute string-lengths cover a distance of only 3/4, this obviously would have been calculated on a monochord.
Zalzal's 49/54 fret results from a division of the string-length between the open string and his 22/27 "middle-finger" fret exactly in half: 1/1 = 54/54, and 22/27 = 44/54, so 1/2 of the string distance between them = 49/54. This fret also has the result of dividing the string-length between the open string and 8/9 (the whole-tone or major-2nd) into 6 equal parts: 1/1 = 54/54, 5/6 of the string distance above that = 49/54, and 1/6 of the string distance above that = 48/54 = 8/9.
Despite the fact that later theorists revised the placement of these frets to render them within a Pythagorean framework, their acoustic gestalts have persisted in music of the "middle-east". This resulted eventually in the controversy over the official adoption by the countries of the Arab world of 24edo ("quarter-tones") in 1932, since 24edo gives good approximations to all of the 11-limit ratios posited by Zalzal.
Zalzal's descending tetrachord-division has the proportion 243 : 256 : 264 : 288 : 294 : 324. Some EDOs which approximate it well are:
- 41edo: degrees 17, 14, 12, 7, 6, 0
- 48edo: degrees 20, 16, 14, 8, 7, 0
- 58edo: degrees 24, 20, 17, 10, 8, 0
- 65edo: degrees 27, 22, 19, 11, 9, 0
- 94edo: degrees 39, 32, 28, 16, 13, 0
Zalzal's fret measurements:
2,3,5,7,11-monzo ---------- ratio --------- string & finger string-length Semitones Ellis's cents [ 5 -2, 0 0 0 > 32 / 9 3.555555556 4 little 3 / 4 9.96 792 [ -3 3, 0 0 0 > 27 / 8 3.375 4 ring 64 / 81 9.06 702 [ 2 2, 0 0 -1 > 36 / 11 3.272727273 4 middle (Zalzal) 22 / 27 8.53 649 [ 0 1, 0 0 0 > 3 / 1 3.0 4 index 8 / 9 7.02 498 [ 4 2, 0 -2 0 > 144 / 49 2.93877551 4 near-index (Zalzal) 49 / 54 6.66 462 [ 3 -1, 0 0 0 > 8 / 3 2.666666667 4 open = 3 little 1 / 1 4.98 294 [ -5 4, 0 0 0 > 81 / 32 2.53125 3 ring 64 / 81 4.08 204 [ 0 3, 0 0 -1 > 27 / 11 2.454545455 3 middle (Zalzal) 22 / 27 3.55 151 [ -2 2, 0 0 0 > 9 / 4 2.25 3 index 8 / 9 2.04 0 [ 2 3, 0 -2 0 > 108 / 49 2.204081633 3 near-index (Zalzal) 49 / 54 1.68 1164 [ 1 0, 0 0 0 > 2 / 1 2.0 3 open = 2 little 1 / 1 0.00 996 [ -7 5, 0 0 0 > 243 / 128 1.8984375 2 ring 64 / 81 11.10 906 [ -2 4, 0 0 -1 > 81 / 44 1.840909091 2 middle (Zalzal) 22 / 27 10.57 853 [ -4 3, 0 0 0 > 27 / 16 1.6875 2 index 8 / 9 9.06 702 [ 0 4, 0 -2 0 > 81 / 49 1.653061224 2 near-index (Zalzal) 49 / 54 8.70 666 [ -1 1, 0 0 0 > 3 / 2 1.5 2 open = 1 little 1 / 1 7.02 498 [ -9 6, 0 0 0 > 729 / 512 1.423828125 1 ring 64 / 81 6.12 408 [ -4 5, 0 0 -1 > 243 / 176 1.380681818 1 middle (Zalzal) 22 / 27 5.58 355 [ -6 4, 0 0 0 > 81 / 64 1.265625 1 index 8 / 9 4.08 204 [ -2 5, 0 -2 0 > 243 / 196 1.239795918 1 near-index (Zalzal) 49 / 54 3.72 168 [ -3 2, 0 0 0 > 9 / 8 1.125 1 open 1 / 1 2.04 0
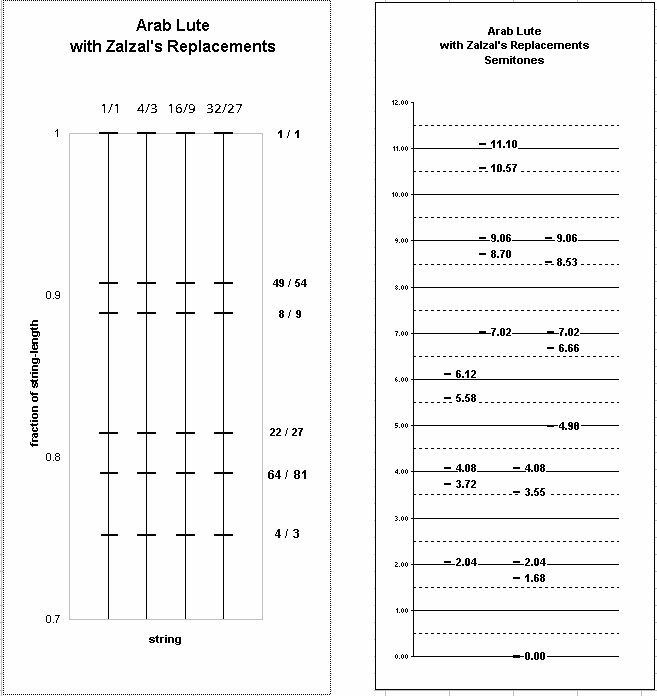
(I use HEWM notation here, in
both the JI and 72edo forms,
for the ratios of 7 and 11.)
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
Abu Nasr al-Farabi (870 - 950 AD) described a lute
fretting which encompassed both the old Pythagorean fretting
and approximations to the two new intervals of Zalzal.
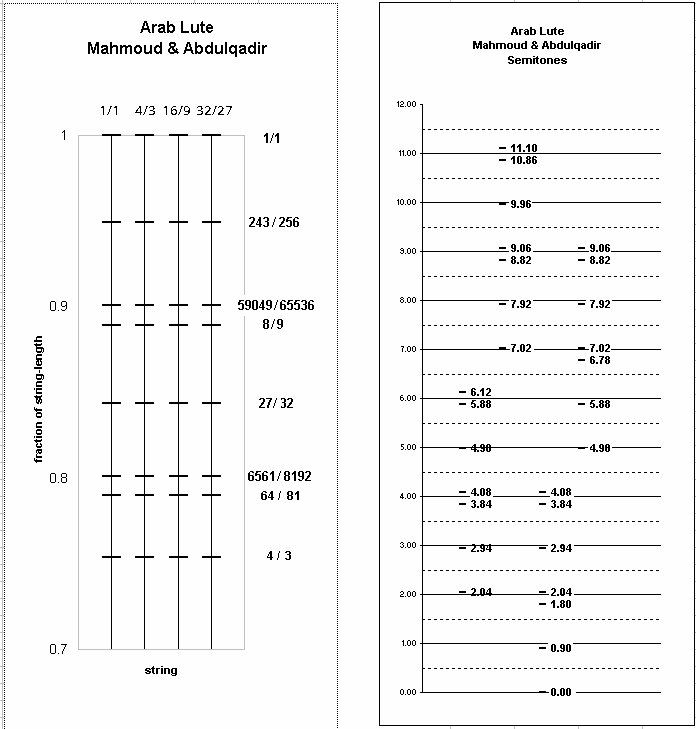
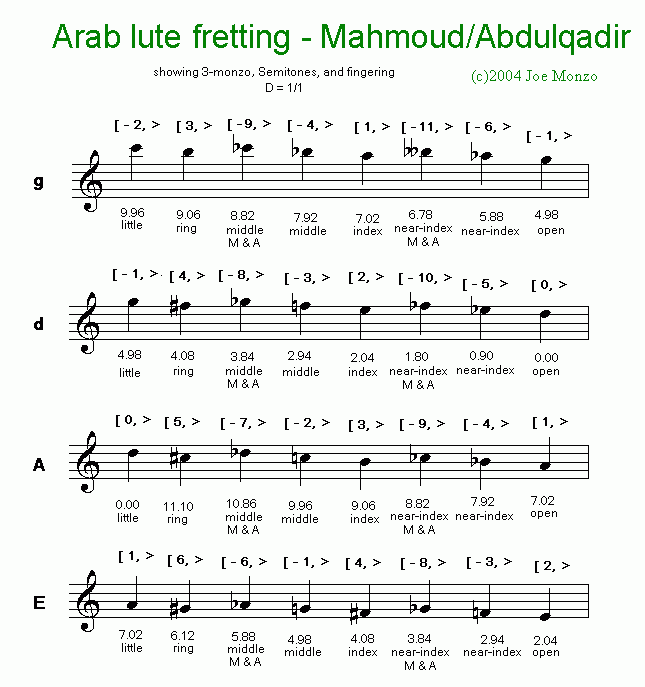
During the 1300s, Mahmoud and Abdulqadir both (independently) reinstated some of the ancient Pythagorean notes, and also moved the placement of Zalzal's frets to nearby notes from an extended Pythagorean chain. The entire system is an 18-tone Pythagorean chain 3(-11 ... +6).
(The "Arabic scale" is always described as a 17-tone scale, as in both Helmholtz and Partch -- see my tabulation of Partch's description. It is not clear to me how one note was lost.)
The adjusted fret placements specified by Mahmoud and Abdulqadir:
2,3,5,7,11-monzo ---------- ratio ---------- string & finger string-length Semitones Ellis's cents [ 5 -2, 0 0 0 > 32 / 9 3.555555556 4 little 3 / 4 9.96 792 [ -3 3, 0 0 0 > 27 / 8 3.375 4 ring 64 / 81 9.06 702 [ 16 -9, 0 0 0 > 65536 / 19683 3.329573744 4 middle (M&A) 6561 / 8192 8.82 678 [ 8 -4, 0 0 0 > 256 / 81 3.160493827 4 middle (ancient) 27 / 32 7.92 588 [ 0 1, 0 0 0 > 3 / 1 3.0 4 index 8 / 9 7.02 498 [ 19 -11, 0 0 0 > 524288 / 177147 2.959621106 4 near-index (M&A) 59049 / 65536 6.78 475 [ 11 -6, 0 0 0 > 2048 / 729 2.809327846 4 near-index (ancient) 243 / 256 5.88 384 [ 3 -1, 0 0 0 > 8 / 3 2.666666667 4 open = 3 little 1 / 1 4.98 294 [ -5 4, 0 0 0 > 81 / 32 2.53125 3 ring 64 / 81 4.08 204 [ 14 -8, 0 0 0 > 16384 / 6561 2.497180308 3 middle (M&A) 6561 / 8192 3.84 180 [ 6 -3, 0 0 0 > 64 / 27 2.37037037 3 middle (ancient) 27 / 32 2.94 90 [ -2 2, 0 0 0 > 9 / 4 2.25 3 index 8 / 9 2.04 0 [ 17 -10, 0 0 0 > 131072 / 59049 2.219715829 3 near-index (M&A) 59049 / 65536 1.80 1177 [ 9 -5, 0 0 0 > 512 / 243 2.106995885 3 near-index (ancient) 243 / 256 0.90 1086 [ 1 0, 0 0 0 > 2 / 1 2.0 3 open = 2 little 1 / 1 0.00 996 [ -7 5, 0 0 0 > 243 / 128 1.8984375 2 ring 64 / 81 11.10 906 [ 12 -7, 0 0 0 > 4096 / 2187 1.872885231 2 middle (M&A) 6561 / 8192 10.86 882 [ 4 -2, 0 0 0 > 16 / 9 1.777777778 2 middle (ancient) 27 / 32 9.96 792 [ -4 3, 0 0 0 > 27 / 16 1.6875 2 index 8 / 9 9.06 702 [ 15 -9, 0 0 0 > 32768 / 19683 1.664786872 2 near-index (M&A) 59049 / 65536 8.82 678 [ 7 -4, 0 0 0 > 128 / 81 1.580246914 2 near-index (ancient) 243 / 256 7.92 588 [ -1 1, 0 0 0 > 3 / 2 1.5 2 open = 1 little 1 / 1 7.02 498 [ -9 6, 0 0 0 > 729 / 512 1.423828125 1 ring 64 / 81 6.12 408 [ 10 -6, 0 0 0 > 1024 / 729 1.404663923 1 middle (M&A) 6561 / 8192 5.88 384 [ 2 -1, 0 0 0 > 4 / 3 1.333333333 1 middle (ancient) 27 / 32 4.98 294 [ -6 4, 0 0 0 > 81 / 64 1.265625 1 index 8 / 9 4.08 204 [ 13 -8, 0 0 0 > 8192 / 6561 1.248590154 1 near-index (M&A) 59049 / 65536 3.84 180 [ 5 -3, 0 0 0 > 32 / 27 1.185185185 1 near-index (ancient) 243 / 256 2.94 90 [ -3 2, 0 0 0 > 9 / 8 1.125 1 open 1 / 1 2.04 0
(Note that, again, regular Pythagorean notation is sufficient for the
frettings of Mahmoud and Abdulqadir.)
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
Lastly, graphs showing all frettings together,
for comparison. (I have also included some Persian frets
which i derived from Ellis's cents measurements in Helmholtz 1954,
but an explanation of that must wait for a future webpage.)
2,3,5,7,11-monzo ---------- ratio ---------- string & finger string-length Semitones Ellis's cents [ 5 -2, 0 0 0 > 32 / 9 3.555555556 4 little 3 / 4 9.96 792 [ -3 3, 0 0 0 > 27 / 8 3.375 4 ring 64 / 81 9.06 702 [ 16 -9, 0 0 0 > 65536 / 19683 3.329573744 4 middle (M&A) 6561 / 8192 8.82 678 [ 2 2, 0 0 -1 > 36 / 11 3.272727273 4 middle (Zalzal) 22 / 27 8.53 649 [ 16 -1, -4 0 -1 > 65536 / 20625 3.17750303 4 middle (Persian) 6875 / 8192 8.01 598 [ 8 -4, 0 0 0 > 256 / 81 3.160493827 4 middle (ancient) 27 / 32 7.92 588 [ 0 1, 0 0 0 > 3 / 1 3.0 4 index 8 / 9 7.02 498 [ 19 -11, 0 0 0 > 524288 / 177147 2.959621106 4 near-index (M&A) 59049 / 65536 6.78 475 [ 4 2, 0 -2 0 > 144 / 49 2.93877551 4 near-index (Zalzal) 49 / 54 6.66 462 [ 23 -10, 0 -2 0 > 8388608 / 2893401 2.899220675 4 near-index (Persian) 964467 / 1048576 6.43 439 [ 11 -6, 0 0 0 > 2048 / 729 2.809327846 4 near-index (ancient) 243 / 256 5.88 384 [ 3 -1, 0 0 0 > 8 / 3 2.666666667 4 open = 3 little 1 / 1 4.98 294 [ -5 4, 0 0 0 > 81 / 32 2.53125 3 ring 64 / 81 4.08 204 [ 14 -8, 0 0 0 > 16384 / 6561 2.497180308 3 middle (M&A) 6561 / 8192 3.84 180 [ 0 3, 0 0 -1 > 27 / 11 2.454545455 3 middle (Zalzal) 22 / 27 3.55 151 [ 14 0, -4 0 -1 > 16384 / 6875 2.383127273 3 middle (Persian) 6875 / 8192 3.03 100 [ 6 -3, 0 0 0 > 64 / 27 2.37037037 3 middle (ancient) 27 / 32 2.94 90 [ -2 2, 0 0 0 > 9 / 4 2.25 3 index 8 / 9 2.04 0 [ 17 -10, 0 0 0 > 131072 / 59049 2.219715829 3 near-index (M&A) 59049 / 65536 1.80 1177 [ 2 3, 0 -2 0 > 108 / 49 2.204081633 3 near-index (Zalzal) 49 / 54 1.68 1164 [ 21 -9, 0 -2 0 > 2097152 / 964467 2.174415506 3 near-index (Persian) 964467 / 1048576 1.45 1141 [ 9 -5, 0 0 0 > 512 / 243 2.106995885 3 near-index (ancient) 243 / 256 0.90 1086 [ 1 0, 0 0 0 > 2 / 1 2.0 3 open = 2 little 1 / 1 0.00 996 [ -7 5, 0 0 0 > 243 / 128 1.8984375 2 ring 64 / 81 11.10 906 [ 12 -7, 0 0 0 > 4096 / 2187 1.872885231 2 middle (M&A) 6561 / 8192 10.86 882 [ -2 4, 0 0 -1 > 81 / 44 1.840909091 2 middle (Zalzal) 22 / 27 10.57 853 [ 12 1, -4 0 -1 > 12288 / 6875 1.787345455 2 middle (Persian) 6875 / 8192 10.05 801 [ 4 -2, 0 0 0 > 16 / 9 1.777777778 2 middle (ancient) 27 / 32 9.96 792 [ -4 3, 0 0 0 > 27 / 16 1.6875 2 index 8 / 9 9.06 702 [ 15 -9, 0 0 0 > 32768 / 19683 1.664786872 2 near-index (M&A) 59049 / 65536 8.82 678 [ 0 4, 0 -2 0 > 81 / 49 1.653061224 2 near-index (Zalzal) 49 / 54 8.70 666 [ 19 -8, 0 -2 0 > 524288 / 321489 1.63081163 2 near-index (Persian) 964467 / 1048576 8.47 643 [ 7 -4, 0 0 0 > 128 / 81 1.580246914 2 near-index (ancient) 243 / 256 7.92 588 [ -1 1, 0 0 0 > 3 / 2 1.5 2 open = 1 little 1 / 1 7.02 498 [ -9 6, 0 0 0 > 729 / 512 1.423828125 1 ring 64 / 81 6.12 408 [ 10 -6, 0 0 0 > 1024 / 729 1.404663923 1 middle (M&A) 6561 / 8192 5.88 384 [ -4 5, 0 0 -1 > 243 / 176 1.380681818 1 middle (Zalzal) 22 / 27 5.58 355 [ 10 2, -4 0 -1 > 9216 / 6875 1.340509091 1 middle (Persian) 6875 / 8192 5.07 303 [ 2 -1, 0 0 0 > 4 / 3 1.333333333 1 middle (ancient) 27 / 32 4.98 294 [ -6 4, 0 0 0 > 81 / 64 1.265625 1 index 8 / 9 4.08 204 [ 13 -8, 0 0 0 > 8192 / 6561 1.248590154 1 near-index (M&A) 59049 / 65536 3.84 180 [ -2 5, 0 -2 0 > 243 / 196 1.239795918 1 near-index (Zalzal) 49 / 54 3.72 168 [ 17 -7, 0 -2 0 > 131072 / 107163 1.223108722 1 near-index (Persian) 964467 / 1048576 3.49 145 [ 5 -3, 0 0 0 > 32 / 27 1.185185185 1 near-index (ancient) 243 / 256 2.94 90 [ -3 2, 0 0 0 > 9 / 8 1.125 1 open 1 / 1 2.04 0
|
|
|
Relevant analyses of Arabic lute-fretting appear on
p 280-284 and 515-517 of Helmholtz 1954; and descriptions
appear on p 370-371, 373, 404, 425, and 430 of Partch 1974.
REFERENCES
al-Kindi (d.873).
Abu Nasr al-Farabi (d.950).
Kitab al-Musiqa al-Kabir [Grand Treatise on Music].
reprint: Gattas 'Abd al-Malik Hashaba, Dar al-katib al-'arabi, Cairo, 1967.
French translation by F.R. d'Erlanger, 1930
Ibn Sina [Avicenna] (d. 1037).
Kitabu a-Sifa.
French translation by F.R. d'Erlanger, 1935.
Auicene perhypatetici philosophi: ac medicorum facile primi opera in luce redacta...
Latin translation published in 1508.
Facsimile edition, Minerva, Frankfurt am Main, 1961.
Safi ad-Din al-Urmawi (d. 1291).
Al-Risala al-Sharafiya fi'l-nisab al-ta'lifiya.
Sezgin, F. (ed.).
Book on the Cyclic Forms of Musical Modes: Kitab al-Adwar
and Treatise dedicated to Sharaf al-Din on Proportions in Musical Composition:
Al-Risala al-Sharafiya fi'l-nisab al-ta'lifiya
by Safi al-Din al-Urmawi Abd al-Mu'mim ibn Ysuf ibn Fakhir (d. 1294 A.D.).
Institute for the History of Arabic-Islamic Science, Ser. C vol. 6, Frankfurt a.M., 1984.
Abdurrahman Jami
Treatise on Music.
Russian translation by V. N. Belyaev, 1960.
Auicene perhypatetici philosophi: ac medicorum facile primi opera in luce redacta...
Latin translation published in 1508.
Facsimile edition, Minerva, Frankfurt am Main, 1961.
Helmholtz, Hermann L.F. von. 1877 (1954).
On the Sensations of Tone as a Psychological basis for the Theory of Music.
reprint by Dover Publications of: 2nd English edition translated by Alexander John Ellis,
based on the 4th German edition of 1877 with extensive notes, foreword and afterword: 1885, of:
Die Lehre von den Tonempfindungen als physiologische Grundlage für die Theorie der Musik.
6th edition, Friedrig Vieweg & Sohn, Braunschweig, 1913, 668 pages. Darmstadt, 1968.
Olms, Hildesheim, 1983.
The 1911 Edition Encyclopaedia, "lute"
Hefny, Mahmoud el. 1931.
Ibn Sinas Musiklehre.
PhD diss., Otto Hellwig, Berlin.
Partch, Harry. 1974.
Genesis of a Music.
University of Wisconsin Press, Madison, 1949.
2nd enlarged edition
Da Capo Press, New York, 1974, 517 pages. Reprint 1979.
(Partch's description tabulated in:
Monzo, Joe. 2001.
An
Examination of Partch's comparison of Equal Temperaments).
Racy, A. J. Arab Music.
Touma, Habib Hassan. 1995.
"Basics of Ratio in Arab Music. The schismatic Permutation of Safiyyuddin al-Urmawi",
Lux Oriente. Festschrift Robert Günther zum 65. Geburtstag.
Gustav Bosse-Verlag, Kassel.
Yahoo tuning list posts, 2002.
Breed, Graham.
message 34993
Wed Feb 27, 2002 1:03 pm
Monzo, Joe.
message 34998
Wed Feb 27, 2002 12:34 pm
Monzo, Joe.
message 35020
Thu Feb 28, 2002 2:46 am
Breed, Graham.
message 35022
Thu Feb 28, 2002 3:12 am
Forster, Cris. 2004. essay
on al-Farabi's lute fretting.
updated:
2000.01.24 -- thanks to Paul Erlich for pointing out errors
2004.07.10 -- entire page completely redone
Please make a donation to help keep Tonalsoft online. A recurring monthly contribution would be apprecitated.
Thank you!