A musical tuning temperament family, discovered and named by Gene Ward Smith.
| family name: | ennealimmal, 72&99 |
| period: | 1/9 octave, or 133 & 1/3 cents. Very nearly 27/25 ratio |
| generator: | 36/35 ratio or 21/20 ratio |
| name: | ennealimmal |
| comma: | ennealimma = 2 / (27/25)^9 = 2,3,5-monzo |1 -27 18> = ~0.861826202 cents |
| prime mapping: | [<9 15 22|, <0 -2 -3|] period, generator |
| 5-limit generator: | 250/243 ratio |
| poptimal generators: | 68/1665-edo, 37/906-edo |
| TOP period: | 133.3322145 cents |
| TOP generator: | 49.0061286 cents |
| TOP mapping: | <1199.98993 1901.97096 2786.29033| cents |
| MOS cardinalities: | 18, 27, 45, 72, 99, 171, 270, 441, 612, 1503, 1665 |
| name: | ennealimmal |
| wedgie: | <<18 27 18 1 -22 -34|| |
| prime mapping: | [<9 15 22 26|, <0 -2 -3 -2|] period, generator |
| poptimal generator: |
43/1053-edo, 61/1494-edo, 49/1200-edo (from the last, exactly 49 cents is a poptimal generator.) |
| TOP period: | 133.3373752 cents |
| TOP generator: | 49.0239856 cents |
| TOP mapping: | <1200.03638 1902.01266 2786.35030 3368.72378| cents |
| TM basis: | {2401/2400, 4375/4374} ratios |
| MOS cardinalities: | 18, 27, 45, 72, 99, 171, 270, 441, 612, 1053 |
Below are screen-shots of Tonescape lattices of ennealimmal-45 tuning, with the syntonic-comma and ennealimma as the two unison-vectors:
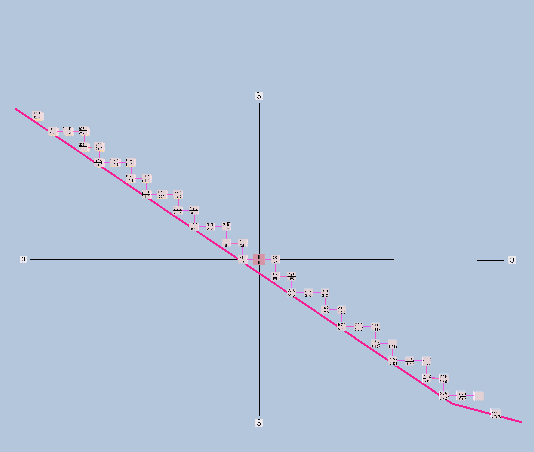
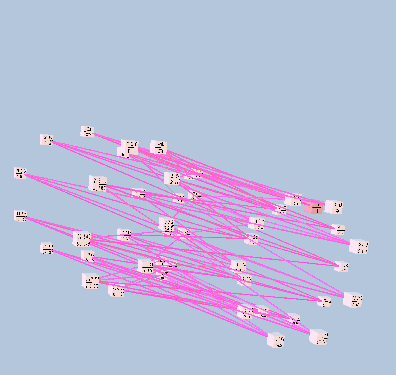
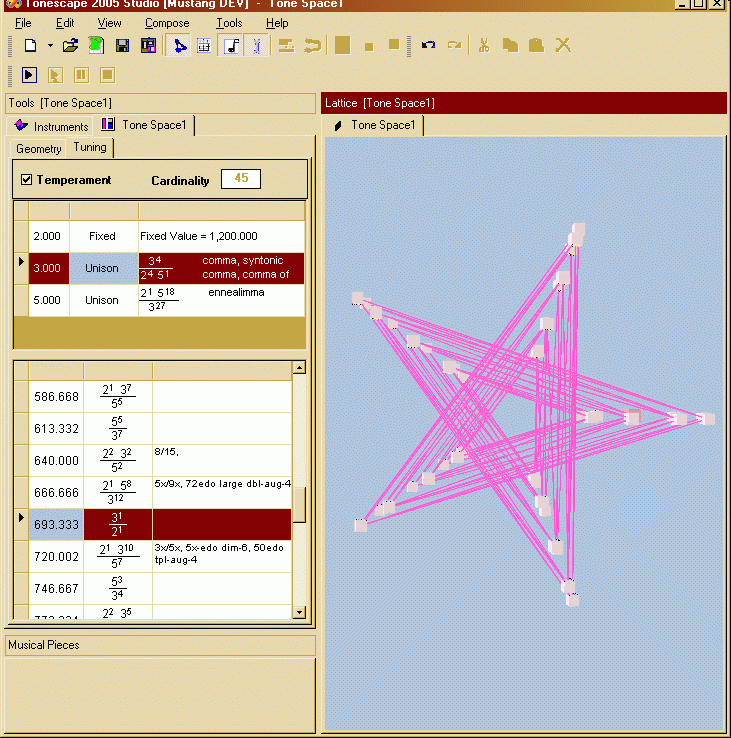
The tonalsoft.com website is almost entirely the work of one person: me, Joe Monzo. Please reward me for my knowledge and effort by choosing your preferred level of financial support. Thank you.