Historical priority of usage of the term "schisma" is by Philolaus (fl. c. 400s BC), as quoted by Boethius, to refer to an interval that is considerably larger than the schisma we normally mean today (see below #2 for that).
Philolaus described the schisma as an integral logarithmic 1/2 of the comma (which today we call the pythagorean-comma):
Philolaus's schisma = comma(1/2)
= (2-19 * 312)(1/2)
= 2-(19/2) * 36 -- thus, 2,3-rational-monzo [ -19/2 6 >
= 11.73 cents.
In its usual modern sense, a term coined by Alexander Ellis in his translation of Helmholtz, On the Sensations of Tone, and originally spelled skhisma. It designates an extremely small interval, just barely discernible to human pitch-detection.
Unqualified, it is the difference between the 51 just "major 3rd" and the 3-8 pythagorean "diminished 4th", and has an interval size of approximately 1/50 Semitone [= ~ 2 cents]:
monzo ratio Semitones ~cents
2 3 5
[ -2 0 1] 5/4 3.86 386.3137139 just "major 3rd"
- [ 13 -8 0] 8192/6561 3.84 384.3599931 pythagorean "diminished 4th"
-------------
[-15 8 1] 32805/32768 0.02 1.953720788 skhisma
The skhisma may also be found as the difference between the pythagorean-comma and the syntonic-comma:
monzo ratio Semitones ~cents
2 3 5
[-19 12 0] 531441/524288 0.235 23.46001038 Pythagorean comma
- [ -4 4 -1] 81/80 0.215 21.5062896 syntonic comma
-------------
[-15 8 1] 32805/32768 0.02 1.953720788 skhisma
Below is a Monzo lattice illustrating the [3 5> prime-factorization of the skhisma:
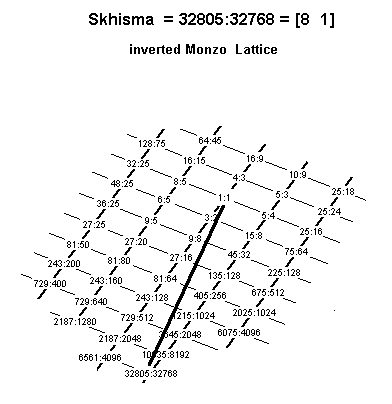
Note that the skhisma is nearly the same size as the grad, the difference between them being only ~0.001280077 (= ~1/781) cent:
2^x 3^y 5^z [ -19/12 1 0 ] grad - [ -15 8 1 ] skhisma ---------------------- [ 161/12 -7 -1 ] difference between grad and skhisma
The standard epimoric approximation to the skhisma is the ratio 887:886 ( = ~1.952886895 cents), whose 2,3,5,443,887-monzo is | -1, 0, 0, -1, 1 > . The tiny difference between them is:
-------- monzo --------
2 3 5 443 887
|-15 8 1 0 0 > = actual skhisma, 32805:32768 ratio
- | -1 0 0 -1 1 > = epimoric approximation, 887:886 ratio
---------------------------
|-14 8 1 1 -1 > = 2076088:2076087 ratio = ~0.000833893 cent ( = ~1/1200 cent)
Tuning treatises before c.1970 often defined the skhisma as the 887:886 ratio without comment on the fact of its being an approximation, particularly in the German literature of c.1850-1950. A good example is in Helmholtz, On the Sensations of Tone....
As no combination of different prime numbers will ever produce ratios which have exactly the same interval size, if cycles of a particular ratio are calculated far enough, very small intervals like this eventually appear between ratios having different sets of prime factors. When these are under consideration, the term "schisma" is qualified with a latin word designating the higher prime, with the assumption that the other prime being compared is a more familiar one, almost always 3.
Thus, we get the "septimal schisma", which is the difference between the 3-14 pythagorean "doubly diminished 8ve" and the 71 harmonic "minor 7th", and has an interval size of approximately 1/26 Semitone:
monzo ratio Semitones ~cents
2 3 7
[23 -14 0] 8388608/4782969 9.73 972.6299879 pythagorean "doubly diminished 8ve"
- [-2 0 1] 7/4 9.69 968.8259065 harmonic "minor 7th"
--------------
[25 -14 -1] 33554432/33480783 0.04 3.804081415 septimal skhisma
Likewise, there is the "nondecimal schisma", which is the difference between the 191 harmonic "augmented 2nd" and the 'standard' 3-3 pythagorean "minor 3rd", and which has an interval size of approximately 1/30 Semitone:
monzo ratio Semitones ~cents
2 3 19
[-4 0 1] 19/16 2.98 297.5130161 harmonic "augmented 2nd"
- [ 5 -3 0] 32/27 2.94 294.1349974 pythagorean "minor 3rd"
---------------
[-9 3 1] 513/512 0.04 3.378018728 nondecimal skhisma
Schismas are a key element in Monzo's concept of bridging. He refers to the three schismas explained here as the 3==5, 3==7, and 3==19 bridges, respectively.
The tonalsoft.com website is almost entirely the work of one person: me, Joe Monzo. Please reward me for my knowledge and effort by choosing your preferred level of financial support. Thank you.