John Coltrane: Giant Steps

analysis of chord progressions
in various tunings

John Coltrane's landmark tune Giant Steps (1960) utilizes a harmonic plan which showcases 12-edo tuning's membership in both the aristoxenean and the augmented temperament families: the former by repetition of the dominant-7th-to-tonic functions, and the latter by the arrangement of tonics as a cycle of major-3rds. The chord progressions are as follows:

LEGEND:

• In the chord symbols, "z" is used to represent the triangle which is commonly used for major; the other two symbols are the usual "x" for dominant and "m" for minor;
• it is assumed that all chords include the 7th, and they may also include the extension 9th and/or 13th;
• the analysis underneath the chord symbols shows how the V-I and II-V-I progressions circulate thru the three keys, which are indicated on the left in brackets.

      Bz Dx  | Gz  Bbx | Ebz | Am  Dx  |
[G]:     V   | I       |     | II  V   |
[Eb]:        |     V   | I   |         |
[B]:  I      |         |     |         |


      Gz Bbx | Ebz F#x | Bz  | Fm  Bbx |
[G]:  I      |         |     |         |
[Eb]:    V   | I       |     | II  V   |
[B]:         |     V   | I   |         |


      Ebz    | Am  Dx  | Gz  | C#m F#x |
[G]:         | II  V   | I   |         |
[Eb]: I      |         |     |         |
[B]:         |         |     | II  V   |


      Bz     | Fm  Bbx | Ebz | C#m F#x |
[G]:         |         |     |         |
[Eb]:        | II  V   | I   |         |
[B]:  I      |         |     | II  V   |
		

Below is another version of the analysis, using more familiar abbreviations for the chord symbols: "M7" = major-7th, "m7" = minor-7th, "7" = dominant-7th:

      BM7 D7  | GM7  Bb7 | EbM7 | Am7  D7  |
[G]:      V   | I        |      | II   V   |
[Eb]:         |      V   | I    |          |
[B]:  I       |          |      |          |


      GM7 Bb7 | EbM7 F#7 | BM7  | Fm7  Bb7 |
[G]:  I       |          |      |          |
[Eb]:     V   | I        |      | II   V   |
[B]:          |      V   | I    |          |


      EbM7    | Am7  D7  | GM7  | C#m7 F#7 |
[G]:          | II   V   | I    |          |
[Eb]: I       |          |      |          |
[B]:          |          |      | II   V   |


      BM7     | Fm7  Bb7 | EbM7 | C#m7 F#7 |
[G]:          |          |      |          |
[Eb]:         | II   V   | I    |          |
[B]:  I       |          |      | II   V   |
		

Giant Steps in 31-edo tuning

31-edo is the first non-12-edo tuning i tried for Giant Steps. I chose 31-edo because it offers low error for prime factors 5 and 7, which are featured prominently in jazz, and its error for 3 is good enough to preserve the approximation to pythagorean root-movement. The arrangement is my own, as i play it on the piano, and the instrument timbre used is acoustic guitar. The original was done in Tonescape^®, and all the other formats were generated automatically from that by Tonescape; below are the downloadable links:

• Tonescape Musical Piece
• MIDI
• Csound unified file (.sco + .orc) -- see also the printout of the Csound output screen
• .wav file

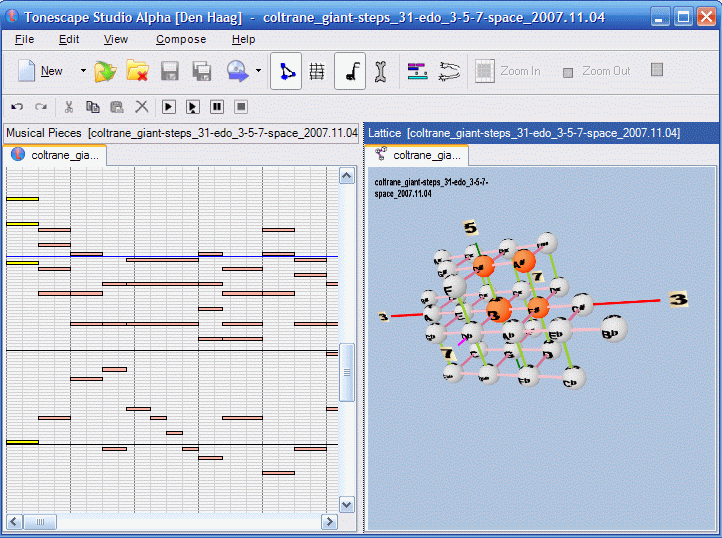
Below is a screenshot of the opening chord being played in Tonescape, with the Lattice using Rectangular Geometry:

Below is the same chord, with the Lattice using Closed Curved crumpled-napkin Geometry:

The chords use the same spelling as in the analysis above, and therefore their progressions do not always follow identical pitch patterns in 31-edo as they do in 12-edo. I plan to make another 31-edo version which does preserve the same pitch patterns, but this will modulate into remote keys which in 12-edo are enharmonic equivalents but in 31-edo are not.