An Euler Genus which contains factors of not only 3 and 5, but also 7. It is thus represented by a 3-dimensional (rather than 2-dimensional) lattice.
John Chalmers gave the following example in his definition of Euler genera, but strictly speaking, since we are noting a difference here, it is an Euler-Fokker genus, because it is 3-dimensional:
[John Chalmers, Divisions of the Tetrachord][For example,] The factors 2n*3*5*7 generate the tones 1/1 35/32 5/4 21/16 3/2 105/64 7/4 15/8 and 2/1 which is also an Octony.
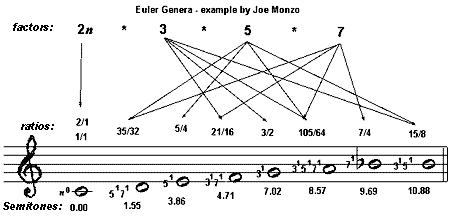
Here is a lattice diagram of the above Euler-Fokker genus, using the 'triangular' convention:
35:32 ---- 105:64
.'/ \'. .'/
5:4 /---\15:8 /
/:\/ \/: /
/ :/\ /\:/
/ 7:4 ---- 21:16
/.' '.\ /.'
1:1 ----- 3:2
The lattice of an Euler-Fokker genus will always bound a cubic or parellelepiped structure.
The tonalsoft.com website is almost entirely the work of one person: me, Joe Monzo. Please reward me for my knowledge and effort by choosing your preferred level of financial support. Thank you.